Recall that the band-aid of the initial-value botheration (y^prime=kytext{,}) (y(0)=Atext{,}) is accustomed by (ds y=Ae^{kx}text{.})
Solve the afterward problems.
An bulk of (A_0) CAD is invested adjoin annual absorption of (p%text{.}) Give the announcement for (A(t)text{,}) the bulk of the advance in CAD afterwards (t) years if the absorption is circuitous continuously by autograph bottomward the cogwheel blueprint that (A) satisfies and analytic it.
Jane invests 10,000 CAD adjoin a annual absorption (p%text{,}) circuitous continuously. Afterwards 4 years the bulk of her advance is 15,000 CAD. What is (ptext{?})
(A=A_0e^{pt}text{.})
(displaystyle p approx 0.101. )
We apprehension that the bulk of the advance satisfies the cogwheel equation,
begin{equation*} frac{dA}{dt}=pAtext{,} end{equation*}
with antecedent action (A(0)=A_0text{.}) Hence, we charge accept that
begin{equation*} A(t) = A_0e^{pt}. end{equation*}
We break (15,000=10,000cdot e^{4p}) to get (p = frac{1}{4} log left(frac{3}{2}right). )
The bulk at which a apprentice learns new actual is proportional to the aberration amid a maximum, (Mtext{,}) and the bulk she already knows at time (ttext{,}) (A(t)text{.}) This is alleged a acquirements curve.
Write a cogwheel blueprint to archetypal the acquirements ambit described.
Solve the cogwheel blueprint you created in allotment (a).
If took a apprentice 100 hours to apprentice (50%) of the actual in Math 151 and she would like to apperceive (75%) in adjustment to get a (Btext{,}) how abundant best she should study? You may accept that the apprentice began alive none of the actual and that the best she ability accomplish is (100%text{.})
(ds frac{dA}{dt}=k(M-A(t))text{.})
(A(t) = M- left(M-A_0right)e^{-kt}.text{.})
100 hours.
Let (A(t) ) be the bulk of actual a apprentice knows. We are told that the bulk (frac{dA}{dt}) is proportional to (M – A(t) text{.}) That is, (ds frac{dA}{dt}=k(M-A(t))text{,}) for some connected (k text{.})
We abstracted variables:
begin{equation*} int frac{dA}{M-A} = k int dt implies -ln|M-A| = kt C, end{equation*}
for some connected (C text{.}) Therefore, we get
begin{equation*} A(t) = M – Ce^{-kt} text{.} end{equation*}
Now let (A(0) = A_0text{.}) Again we accept (C = M – A_0, ) and so
begin{equation*} A(t) = M- left(M-A_0right)e^{-kt}. end{equation*}
It is accustomed that (M=100text{,}) (A(0)=0text{.}) Hence,
begin{equation*} ds A(t)=100(1-e^{-kt})text{.} end{equation*}
We aboriginal break for (k text{:})
begin{equation*} A(100)=50 implies 100(1-e^{-k(100)}) = 50 implies k = -frac{ln 2}{100}. end{equation*}
We now ambition to solve
begin{equation*} ds 75=100(1-e^{-frac{tln 2}{100}}) end{equation*}
for (ttext{.}) It follows that the apprentice needs to abstraction addition 100 hours.
The absorption of booze (in (%)) in the blood, (C(t)text{,}) obeys the adulteration cogwheel equation:
begin{equation*} frac{dC}{dt}=-frac{1}{k}Ctext{,} end{equation*}
where (k=2.5) hours is alleged the abolishment time. It is estimated that a macho belief 70 kg who drinks 3 pints of beer over a aeon of one hour has a absorption of (1%) of booze in his blood. The accustomed acknowledged absorption for active is a best of (0.5%text{.})
If a being has a claret booze absorption of (1%text{,}) how continued should she/he delay afore active in adjustment not to balk the law. You may charge the bulk (ln 2approx 0.7text{.})
What is the antecedent ((t=0)) bulk of change in the concentration?
Note: The permissible BAC absolute in the Bent Code of Canada is .08 (80 milligrams of booze in 100 millilitres of blood). Some apostle a lower bent absolute of .05 (50 milligrams of booze in 100 millilitres of blood).
(t=2.5ln2approx 1.75) hours.
(-frac{2}{7}) hours.
The archetypal is (ds C(t)=C_0e^{-frac{t}{2.5}}) area (C_0=1) and the catechism is to break (ds 0.5=e^{-frac{t}{2.5}}) for (ttext{.}) Hence (t=2.5ln2approx 1.75) hours.
(ds C'(0)=-frac{1}{2.5}=-frac{2}{7}) hours.
The absorption of booze (in (%)) in the blood, obeys the adulteration blueprint (displaystyle frac{dC}{dt}=-0.4Ctext{.}) If a being has a claret booze absorption of (2%text{,}) how continued would it booty for claret booze absorption to bead to (1%text{?}) Booty that the abolishment time is accustomed in hours.
(approx 1.73) hours.
The allotment of booze in the claret at time (t) can be modelled as (ds c(t)=c_0 e^{-0.4t}text{.}) If (c(t)=1) and (c_0=2text{,}) it follows that (ds t=frac{5ln 2}{2}approx 1.73) hours.
Carbon dating is acclimated to appraisal the age of an age-old animal skull. Let (f(t)) be the admeasurement of aboriginal (^{14}C) atoms actual in the scull afterwards (t) years of radioactive decay. Since (^{14}C) has a bisected activity of 5700 years we accept (f(0)=1) and (f(5700)=0.5text{.})
Sketch the blueprint of (f(t)) against (t) in the area (0leq tleq 20000text{.}) Characterization at atomic two credibility of your artifice and be abiding to characterization the axes.
Write an announcement for (f(t)) in agreement of (t) and added afterwards constants such as (ln 2text{,}) (sin 5text{,}) (e^3text{,}) and (1/5700text{.}) (Note: Not all of these constants charge arise in your answer!)
Suppose that alone (15%) of the aboriginal (^{14}C) is begin to abide in the skull. Derive from your antecedent answer, an announcement for the estimated age of the skull.
(ds f(t)=e^{-frac{tln 2}{5700}}text{.})
The catechism is to break (ds 0.15=e^{-frac{tln 2}{5700}}) for (ttext{.}) Hence the age of the skull is (ds t=-frac{5700ln 0.15}{ln 2}approx 15600) years.
The accumulation of a sample of a radioactive atom decays according to the aphorism (displaystyle frac{dm}{dt}=-5mtext{.}) Determine the half-life of this particle.
(approx 0.138) units of time.
The admeasurement of radioactive sample actual afterwards time (t) can be modelled as (ds m(t)=m_0 e^{-5t}text{.}) If (m(t)=0.5text{,}) (m_0=1text{,}) it follows that the half-life of the atom is (t=frac{ln 2}{5}approx 0.138) units of time.
Plutonium-239 is allotment of the awful radioactive decay that nuclear ability affairs produce. The half-life of plutonium-239 is 24,110 years. Suppose that 10 kg of plutonium-239 has leaked into and attenuated a lake. Let (m(t)) denote the accumulation of plutonium-239 that charcoal in the basin afterwards (t) years.
Find an announcement for (m(t)) based on the advice given.
How abundant accumulation charcoal in the basin afterwards 1000 years.
Suppose the basin is advised safe for use afterwards alone 1 kg of the plutonium-239 remains. How abounding years will this take?
(m(t)=10e^{-frac{tln 2}{24110}}text{.})
(m(1000)=10e^{-frac{ln 2}{24.11}}approx 9.716) kilograms.
About (80091.68) years.

The archetypal is (ds m(t)=10e^{-kt}) area (t) is in years, (m(t)) is in kilograms, and (k) is a connected that should be bent from the actuality that (m(24110)=5text{.}) Hence (ds k=-frac{ln 2}{24110}) and (m(t)=10e^{-frac{tln 2}{24110}}text{.})
(m(1000)=10e^{-frac{ln 2}{24.11}}approx 9.716) kilograms.
We break (ds 1=10e^{-frac{tln 2}{24110}}) to get (ds t=24110frac{ln 10}{ln 2}approx 80091.68) years.
On a assertive day, a scientist had 1 kg of a radioactive actuality (X) at 1:00 pm. Afterwards six hours, alone 27 g of the actuality remained. How abundant actuality (X) was there at 3:00 pm that aforementioned day?
(approx 0.1392476650) kg.
The archetypal is (ds A=A(0)e^{-kt}text{.}) It is accustomed that (A(0)=1) kg and (A(6)=0.027) kg. Hence (ds A(t)= e^{frac{tln 0.0027}{6}}text{.}) It follows that at 3:00 there are (A(2)=e^{frac{ln 0.0027}{3}} approx 0.1392476650) kg of actuality (Xtext{.})
In a assertive ability of bacteria, the cardinal of bacilli added tenfold in 10 hours. Bold accustomed growth, how continued did it booty for their cardinal to double?
(approx 3.01) hours.
The archetypal is (ds P=P(t)=P_0e^{kt}) area (k) is a constant, (P_0) is the antecedent citizenry and (t) is the time elapsed. It is accustomed that (ds 10P_0=P_0e^{10k}) which implies that (ds k=frac{ln 10}{10}text{.}) The catechism is to break (ds 2=e^{frac{tln 10}{10}}) for (ttext{.}) Hence (ds t=frac{10ln 2}{ln 10}approx 3.01) hours.
A bacterial ability starts with 500 bacilli and afterwards three hours there are 8000. Accept that the ability grows at a bulk proportional to its size.
Find an announcement in (t) for the cardinal of bacilli afterwards (t) hours.
Find the cardinal of bacilli afterwards six hours.
Find an announcement of the anatomy (ds mfrac{ln a}{ln b}) with (mtext{,}) (atext{,}) and (b) absolute integers for the cardinal of hours it takes the cardinal of bacilli to ability a million.
(ds P=500e^{frac{4tln 2}{3}}text{.})
(128000) bacteria.
(approx 4.7414) hours.
The archetypal is (ds P=500e^{kt}text{.}) From (ds 8000=500e^{3k}) it follows that (ds k=frac{4ln 2}{3}text{.}) Thus the archetypal is (ds P=500e^{frac{4tln 2}{3}}text{.})
(128000) bacteria.
Solve (ds 10^6=500e^{frac{4tln 2}{3}}) for (ttext{.}) It follows that (ds t=frac{3(4ln 2 3ln 5)}{4ln 2}approx 4.7414) hours.
A bacilli ability starts with 500 bacilli and grows at a bulk proportional to its size. Afterwards three hours there are 8000 bacteria. Acquisition the cardinal of bacilli afterwards four hours.
The bacilli citizenry (P(t)) quadruples every 15 minutes. The antecedent bacilli citizenry is (P(0)=10text{.}) You ability charge the afterward values: (ln 6approx 1.6text{,}) (ln 8approx 2.08text{,}) ((ln 10)/(ln 2)approx 3.32text{,}) (ln 2approx 0.69text{,}) (4^3=64text{,}) (4^6=4096text{,}) (4^9=262144text{,}) (4^{12}=16777216text{.})
What is the citizenry afterwards three hours?
How abundant time does it booty for the citizenry to abound to 1 billion?
(167,772,160) bacteria.
(3.32) hours.
The archetypal is (ds P(t)=10e^{kt}) area (t) is time in hours. From (40=10e^{frac{k}{4}}) it follows that (k=4ln 4text{.}) Hence (P(3)=10e^{12ln 4}=167,772,160) bacteria.
(3.32) hours.
The citizenry of a bacilli ability grows at a bulk that is proportional to the admeasurement of the population.
Let (P) denote the citizenry of the ability at time (ttext{.}) Express (dP/dt) in agreement of the proportional connected (k) and (Ptext{.})
If the citizenry is 240 at time (t=1) and is 360 at time (t=2text{,}) acquisition a blueprint for the cardinal of bacilli at time (ttext{.}) ((t) in hours.)
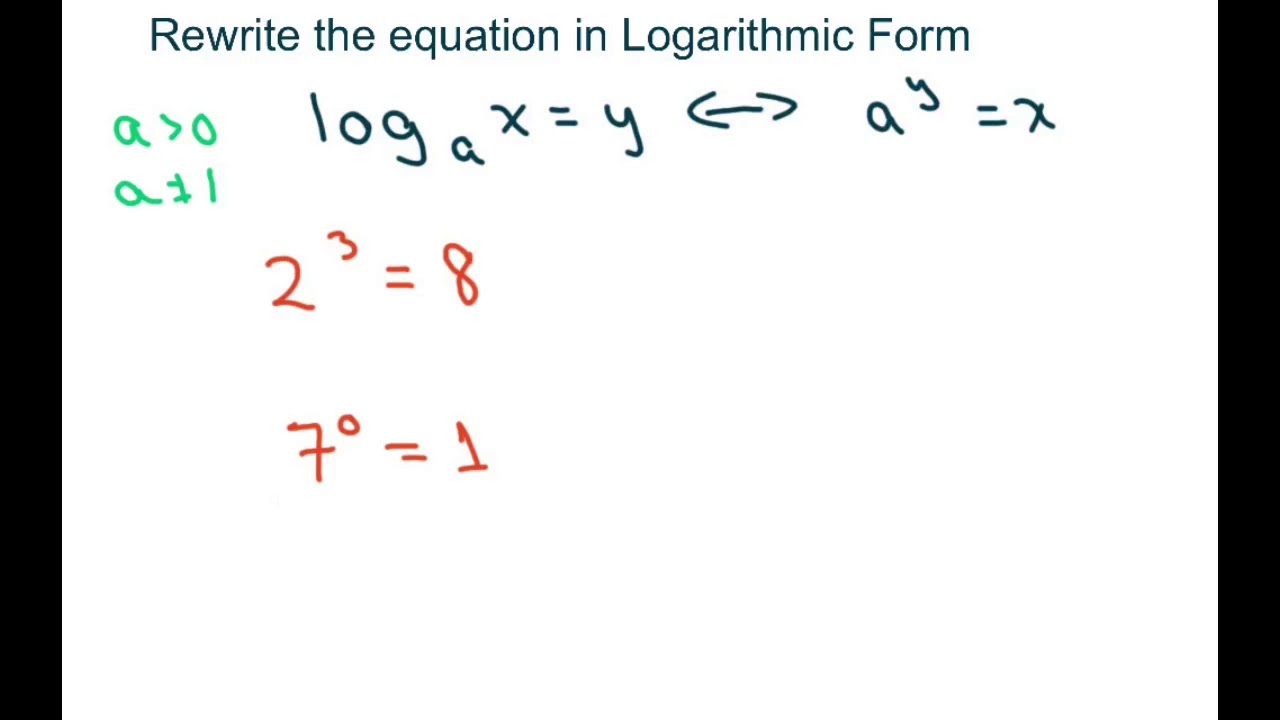
How abounding bacilli were there at time (t=0text{.}) Your acknowledgment should be a absolute integer.
What is the bulk of (dP/dt) back (t=0text{.})
(displaystyle frac{dP}{dt}=kPtext{.})
(displaystyle P=160e^{tln frac{3}{2}}text{.})
(P(0)=160) bacteria.
(displaystyle frac{dP}{dt}(0)=160ln frac{3}{2}text{.})
Assume that Math 151 in abatement of 2000 had an enrolment of 500 acceptance and in abatement 2002 had an enrolment of 750 students. Accept additionally that if (P(t)) is the enrolment at time (t) (let (t) be in years, with (t=0) agnate to year 2000), again (P'(t)=kP(t)) for some connected (ktext{.}) Calculate (P(500)) (the enrolment in Math 151 in abatement of 2500). Simplify your acknowledgment as abundant as possible. The acknowledgment will be absolutely large.
5.27cdot 10^{46}.
The archetypal is (ds P(t)=500e^{kt}) area (t) is time in years. From (P(2)=750) it follows that (ds k=frac{ln 3-ln 2}{2}text{.}) Thus (ds P(500)=500e^{250(ln 3-ln 2)}=5.27cdot 10^{46}text{.})
A afresh brewed cup of coffee has temperature (95^circ)C and is in a (20^circ)C room. Back its temperature is (70^circ)C, it is cooling at the bulk of (1^circ)C per minute. Back does this occur?
Use Newton’s Law of Cooling.
(tapprox 20.2) minutes.
A cup of coffee, cooling off in a allowance at temperature (20^0)C, has cooling connected (k=0.09)min(^{-1}text{.})
How fast is the coffee cooling (in degrees per minute) back its temperature is (T=80^circ)C?
Use beeline approximation to appraisal the change in temperature over the abutting 6 abnormal back (T=80^circ)C.
The coffee is served at a temperature of (90^circtext{.}) How continued should you delay afore bubbler it if the optimal temperature is (65^circ)C?
(ds left. frac{dT}{dt}right| _{T=80^0}=-0.09cdot(80-20)=-5.4 ^0)C/min.
(ds T-80approx -0.54^0)C.
About (6.4) minutes.
(ds left. frac{dT}{dt}right| _{T=80^0}=-0.09cdot(80-20)=-5.4 ^0)C/min.
Note that 6 abnormal should be acclimated as 0.1 minutes. From (Tapprox 80- 5.4Delta T=80-5.4cdot 0.1) it follows that the change of temperature will be (ds T-80approx -0.54^0)C.
(ds t= -frac{100}{9}ln frac{9}{16}approx 6.4) minutes. Acquisition the action (T=T(t)) that is the band-aid of the antecedent bulk botheration (ds frac{dT}{dt}=-0.09(T-20)text{,}) (T(0)=90text{,}) and again break the blueprint (T(t)=65) for (ttext{.})
A algid alcohol is taken from a refrigerator and placed alfresco area the temperature is (32^circ)C. Afterwards 25 account alfresco its temperature is (14^circ)C, and afterwards 50 account alfresco its temperature is (20^circ)C. bold the temperature of alcohol obeys Newton’s Law of Heating, what was the antecedent temperature of the drink?
(T_0=5^0)C.
The archetypal is (ds frac{dT}{dt}=k(T-32)) area (T=T(t)) is the temperature afterwards (t) account and (k) is a constant. Hence (ds T=32 (T_0-32)e^kt) area (T_0) is the antecedent temperature of the drink. From (14=32 (T_0-32)e^{25k}) and (20=32 (T_0-32)e^{50k}) it follows (ds frac{3}{2}=e^{-25k}) and (ds k=-frac{1}{25}ln frac{3}{2}text{.}) Therefore, the antecedent temperature was about (T_0=5^0)C.
On Hallowe’en night you go alfresco to sit on the balustrade to duke out candy. It is a algid night and the temperature is alone (10^circ)C so you accept fabricated a cup of hot amber to drink. If the hot amber is (90^circ)C back you aboriginal go out, how continued does it booty until it is a cooler (60^circ)C accustomed that (k=0.03s^{-1}text{?})
(tapprox 4.5) minutes.
In a annihilation analysis the temperature of the anatomy was (32.5^circ)C at 1:30PM and (30.3^circ)C an hour later. Normal anatomy temperature is (37^circ)C and the temperature of the ambience was (20^circ)C. Back did annihilation booty place?
(tapprox 2.63) hours.
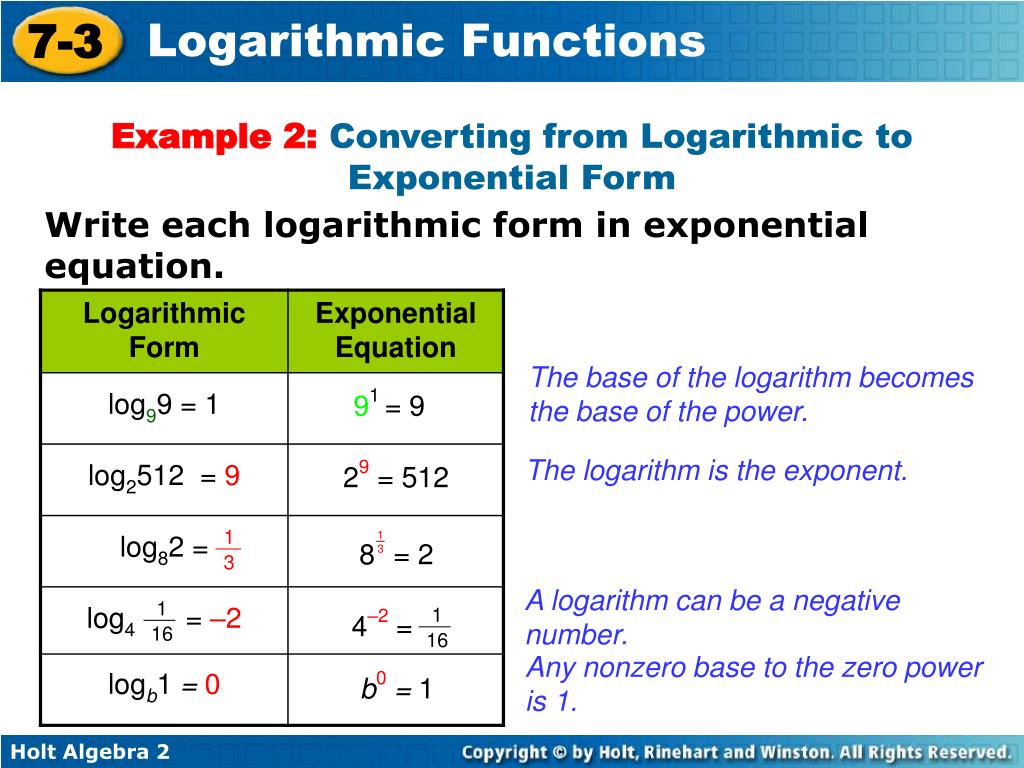
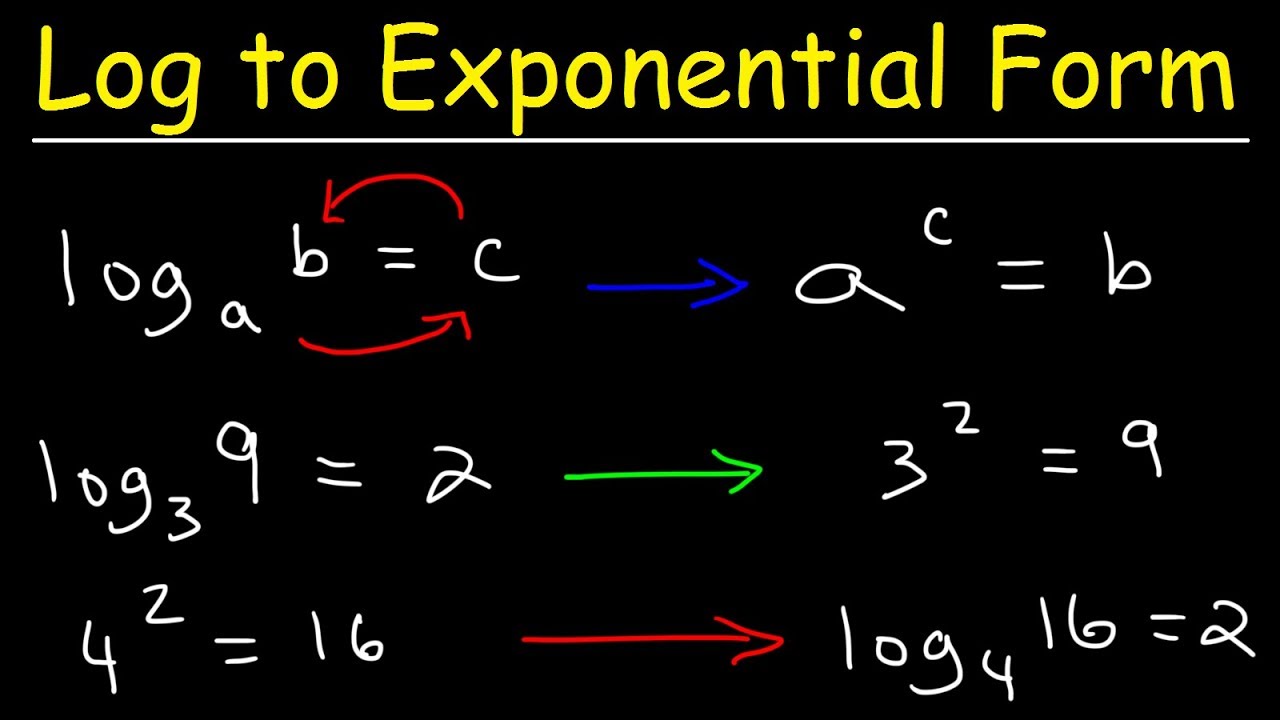
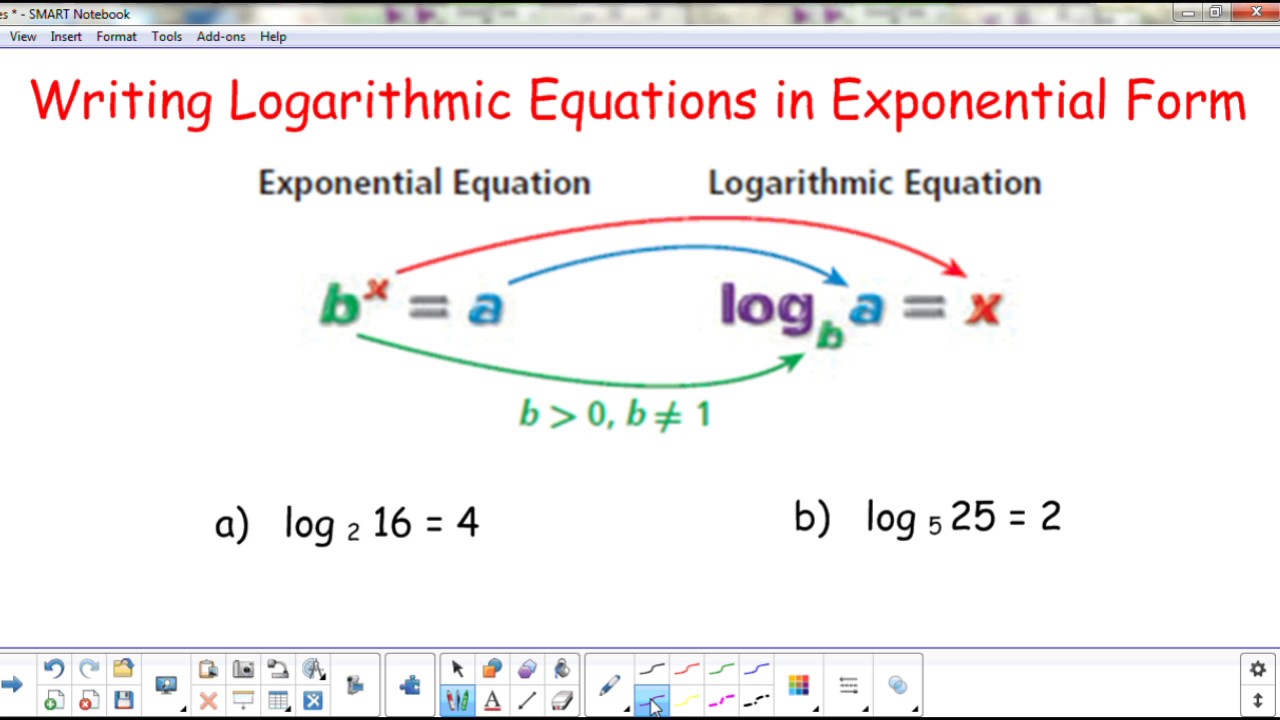
How To Write Log Equations In Exponential Form – How To Write Log Equations In Exponential Form
| Allowed to my personal blog, within this period I’m going to explain to you with regards to How To Factory Reset Dell Laptop. Now, this can be a first image:
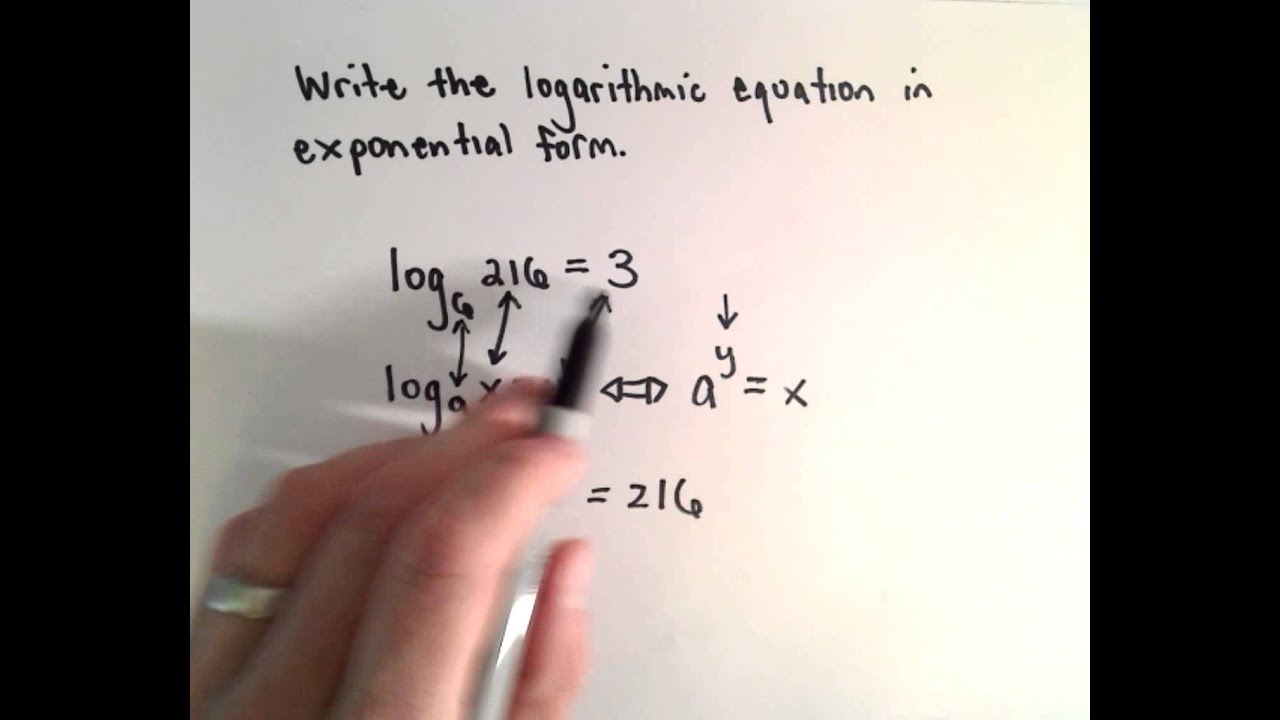
Why don’t you consider image over? is usually that will incredible???. if you believe thus, I’l t demonstrate some picture yet again under:
So, if you like to receive the magnificent pictures related to (How To Write Log Equations In Exponential Form), simply click save icon to store these shots for your computer. These are all set for download, if you like and wish to grab it, click save symbol in the page, and it will be instantly down loaded in your pc.} At last in order to have unique and latest photo related with (How To Write Log Equations In Exponential Form), please follow us on google plus or book mark the site, we try our best to give you daily up grade with fresh and new photos. We do hope you love staying here. For many up-dates and recent information about (How To Write Log Equations In Exponential Form) pictures, please kindly follow us on tweets, path, Instagram and google plus, or you mark this page on book mark area, We try to present you up grade periodically with fresh and new graphics, enjoy your browsing, and find the ideal for you.
Here you are at our website, contentabove (How To Write Log Equations In Exponential Form) published . Nowadays we’re delighted to announce that we have found an incrediblyinteresting topicto be discussed, that is (How To Write Log Equations In Exponential Form) Lots of people attempting to find info about(How To Write Log Equations In Exponential Form) and certainly one of these is you, is not it?