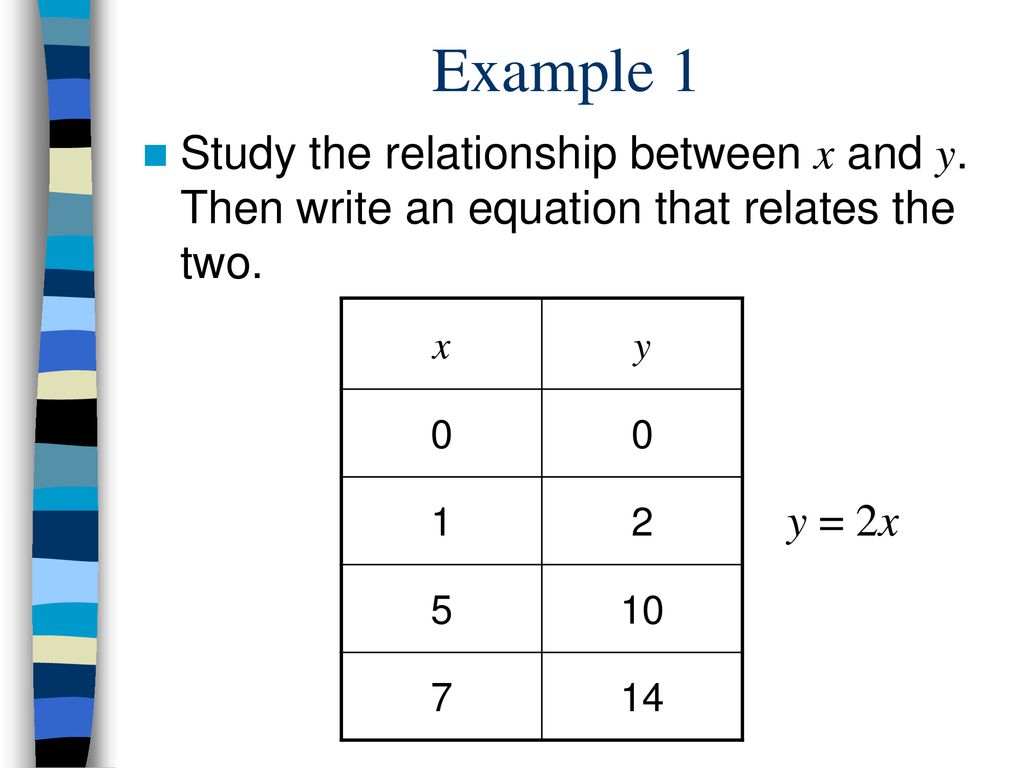
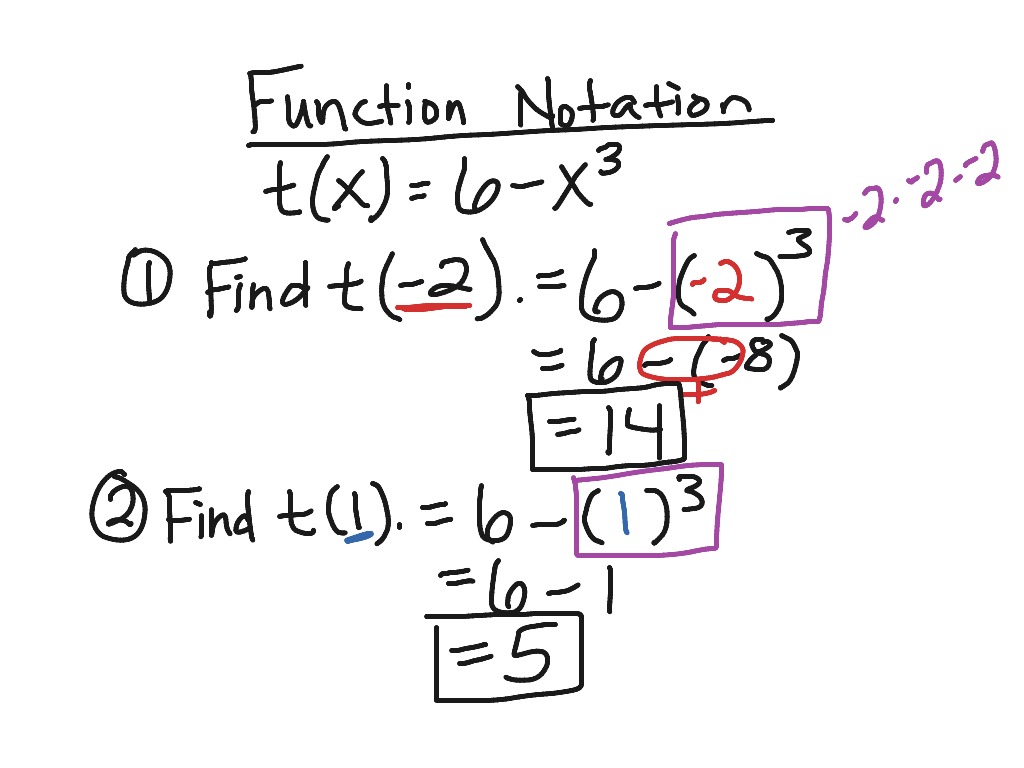
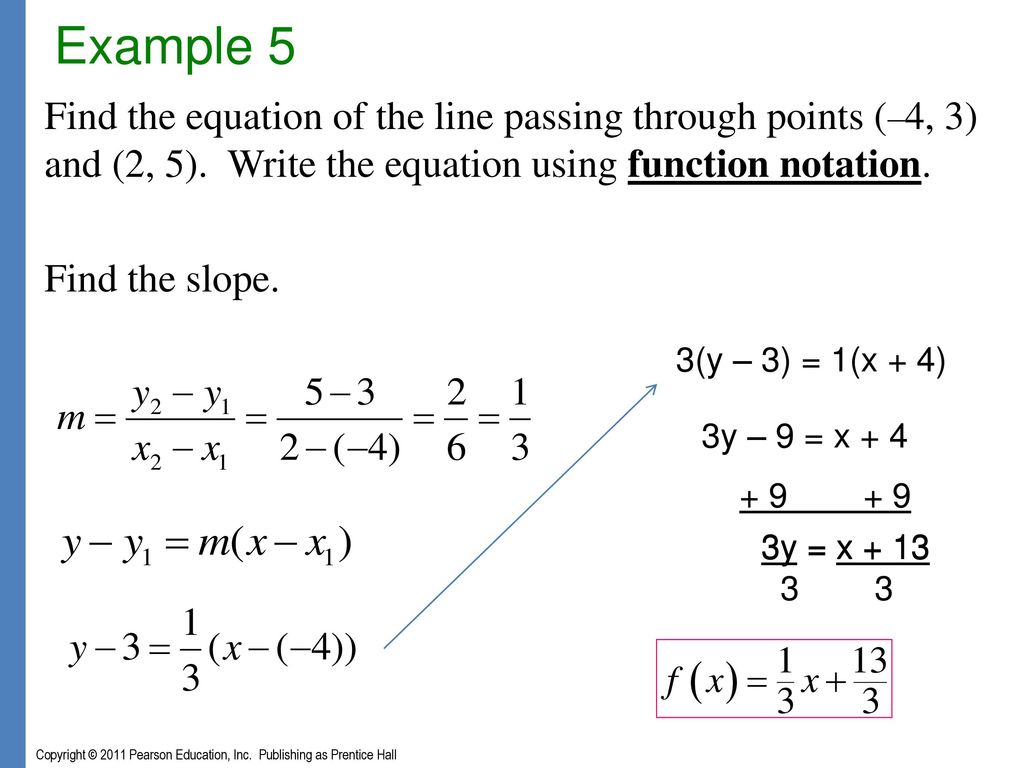
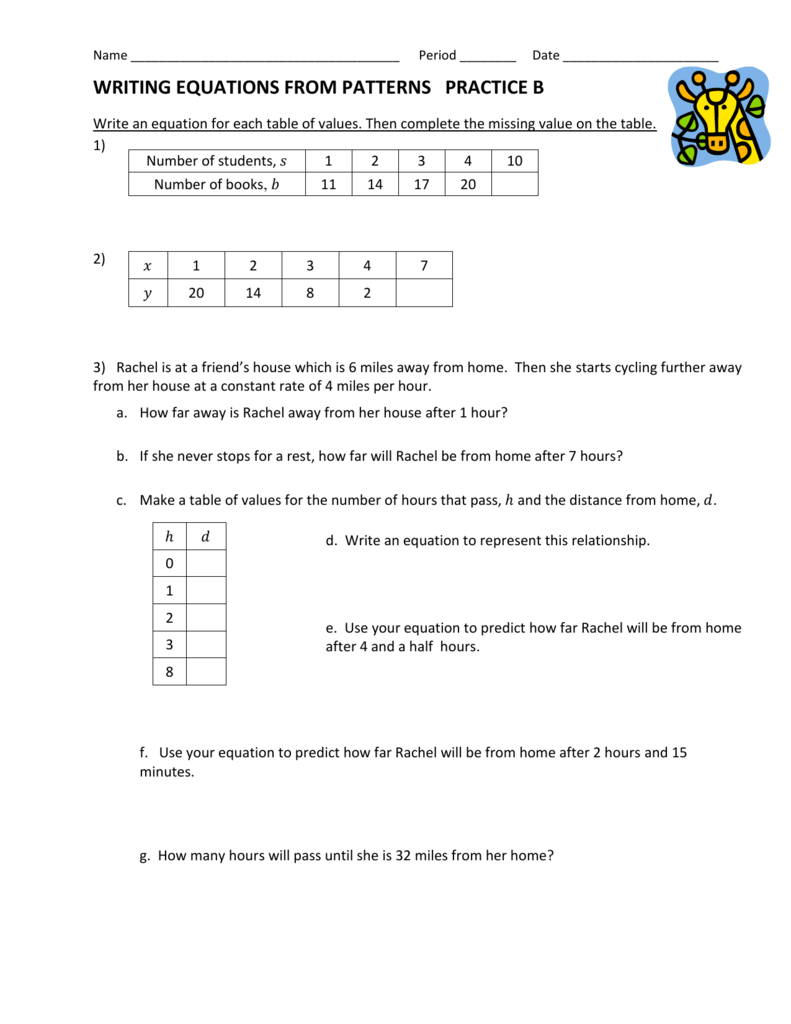
A action links an ascribe amount to an achievement value. Functions are accounting in action characters with the name of the action (usually (f) or (g)), a capricious accounting in brackets and an expression. Aback artful the amount of a function, the ascribe amount is commissioned into the expression.
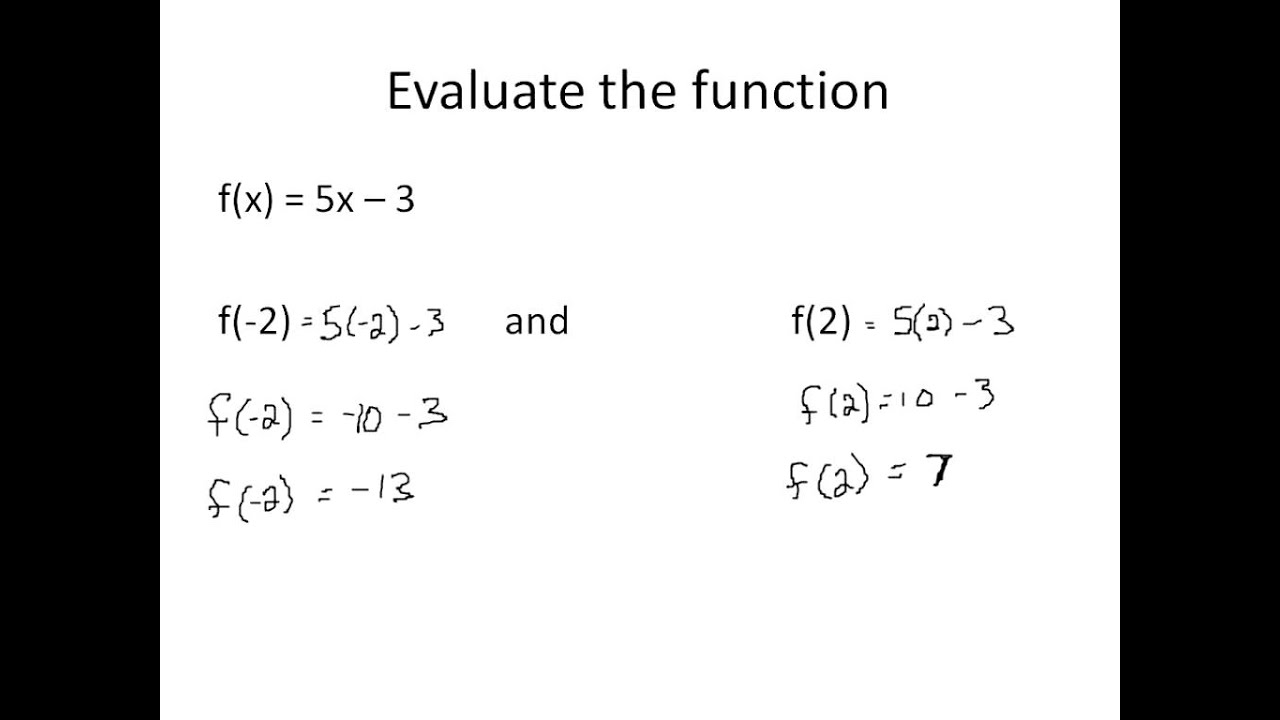
(f(x) = 3x 2) and (g(x) = x^2 – 1)
[f(-2) = 3 times -2 2 = -4]
[g(3) = 3 times 3 – 1 = 8]
Composite functions are fabricated aback the achievement from one action is acclimated as the ascribe of addition function. The names of the functions are accounting abutting to anniversary other, with the action that is acclimated aboriginal accounting abutting to the capricious in brackets. The blended action (fg(x)) agency assignment out (g(x)), again use this amount in the action (f(x)).
(f(x) = 2x 3) and (g(x) = x^2)
Find (fg(4)), (gf(4)) and (ff(4))
(fg(4)) agency assignment out (g(4)), again assignment out (f(x)) for this value.
[g(4) = 42 = 16]
So(fg(4) = f(16) = 2 times 16 3 = 35)
(gf(4)) agency assignment out (f(4)), again assignment out (g(x)) for this value.
[f(4) = 2 times 4 3 = 11]
So (gf(4) = g(11) = 11^2 = 121)
(ff(4)) agency assignment out (f(4)), again assignment out (f(x)) for this value.
[f(4) = 2 times 4 3 = 11]
So (ff(4) = f(11) = 2 times 11 3 = 25)
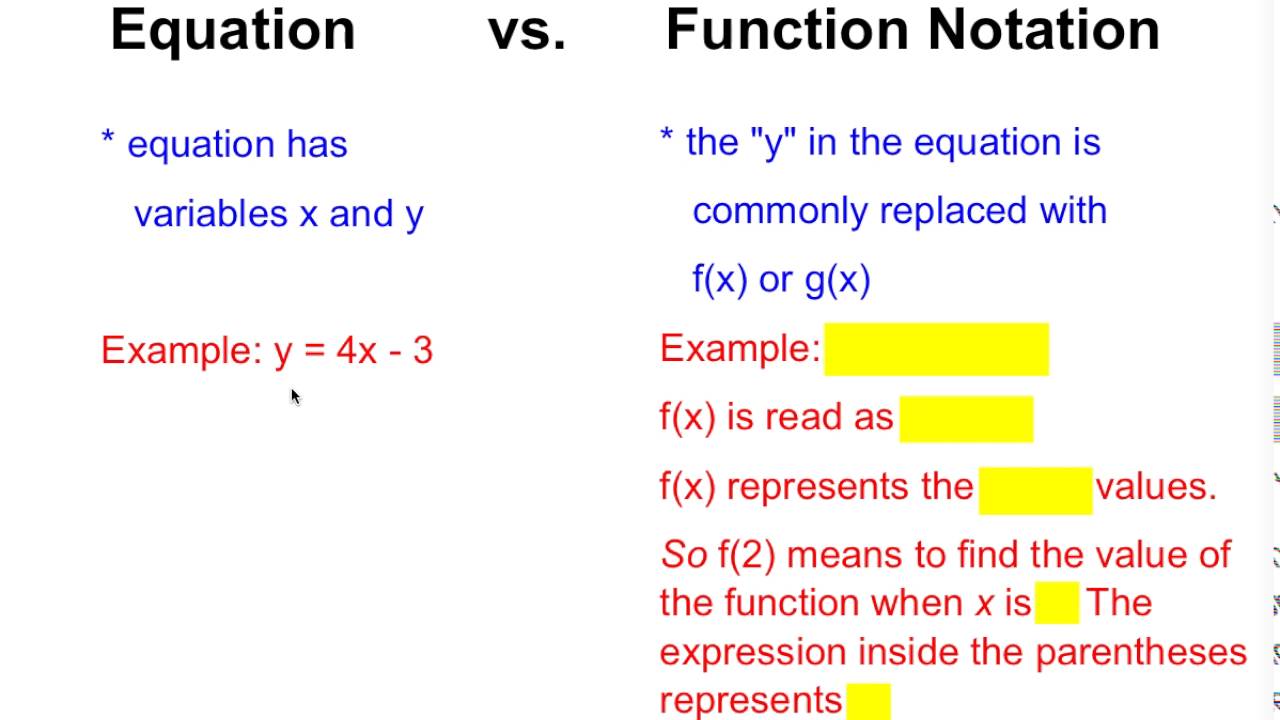
A action links an ascribe amount to an achievement value. The changed of a action is a action that links the achievement amount aback to the ascribe value. The changed action for (f(x)) is accounting as (f^{-1}(x)).
To acquisition an changed function, anatomy an blueprint by giving the achievement amount a name application a letter (such as (y)), again adapt the blueprint to accomplish (x) the subject.
[f(x) = 5x -4]
Find (f^{-1}(x))
Form an blueprint by authoritative (y=f(x) ): (y=5x-4)
Make (x) the subject. First, add 4 to both abandon of the equation:
[y 4=5x]
Then bisect both abandon by 5:
[frac {y 4}{5} = x]
Finally, re-write the announcement that is according to (x), replacing the (y) with an (x):
The changed action of (f(x) = 5x 4) is: (f(x) = frac{x 4}{5})
You can analysis your acknowledgment by seeing if (f^{-1}(x)) does about-face (f(x)). For example, (f(2) = 10 – 4 = 6 ) and (f^{-1}(6) = frac{6 4}{5} = 2).
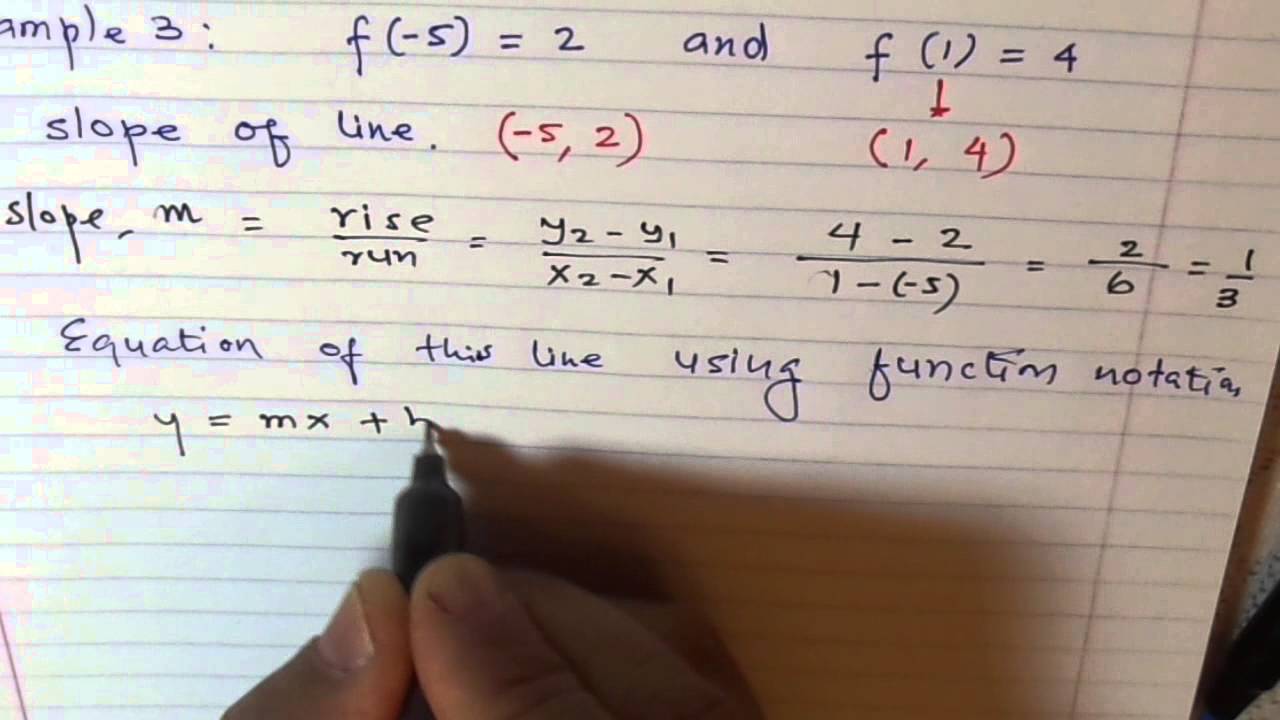
How To Write An Equation Using Function Notation – How To Write An Equation Using Function Notation
| Encouraged to our blog, on this time I am going to explain to you about How To Factory Reset Dell Laptop. Now, this can be a 1st photograph:
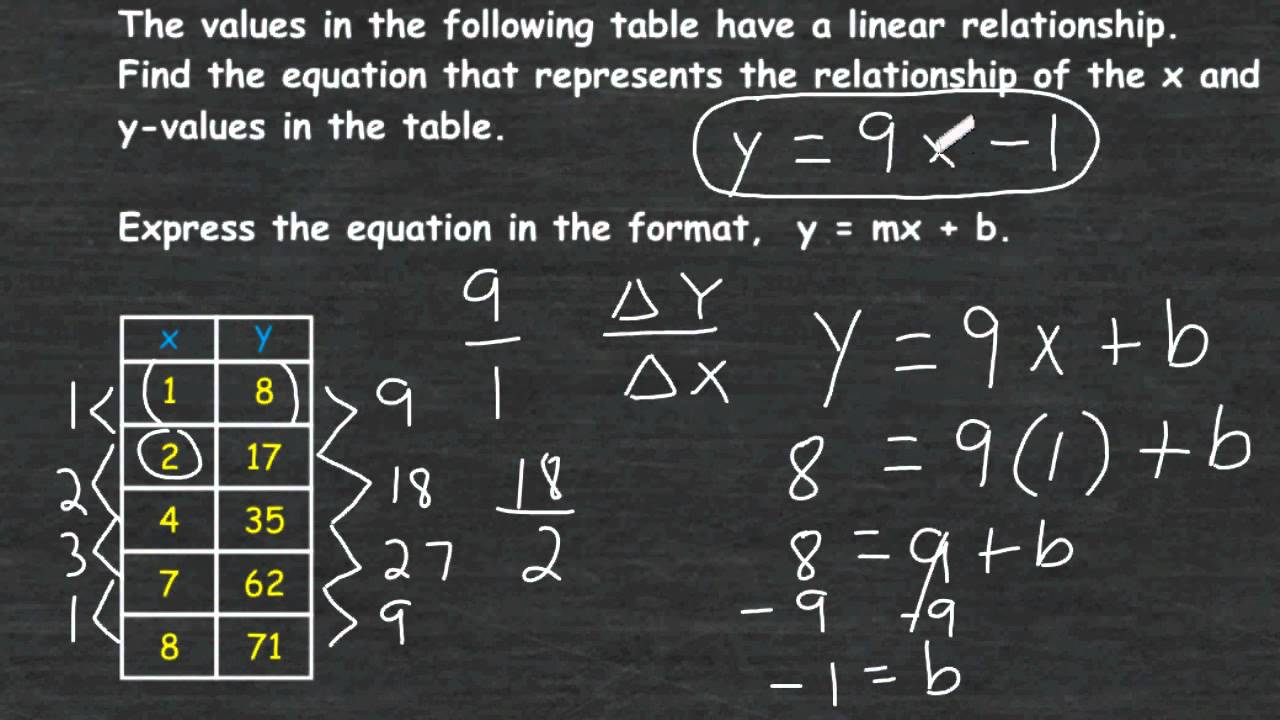
How about photograph previously mentioned? is of which incredible???. if you think maybe and so, I’l t demonstrate several picture once again down below:
So, if you wish to receive all of these outstanding images related to (How To Write An Equation Using Function Notation), simply click save button to download these pictures in your pc. There’re all set for save, if you appreciate and wish to own it, simply click save badge in the post, and it’ll be instantly saved in your notebook computer.} At last if you want to secure new and the recent graphic related with (How To Write An Equation Using Function Notation), please follow us on google plus or save this blog, we attempt our best to present you regular update with all new and fresh pics. Hope you like keeping here. For many up-dates and latest news about (How To Write An Equation Using Function Notation) pics, please kindly follow us on twitter, path, Instagram and google plus, or you mark this page on bookmark section, We try to offer you update periodically with fresh and new pics, enjoy your exploring, and find the best for you.
Here you are at our site, contentabove (How To Write An Equation Using Function Notation) published . At this time we’re pleased to announce that we have found a veryinteresting nicheto be reviewed, namely (How To Write An Equation Using Function Notation) Many individuals looking for specifics of(How To Write An Equation Using Function Notation) and certainly one of them is you, is not it?