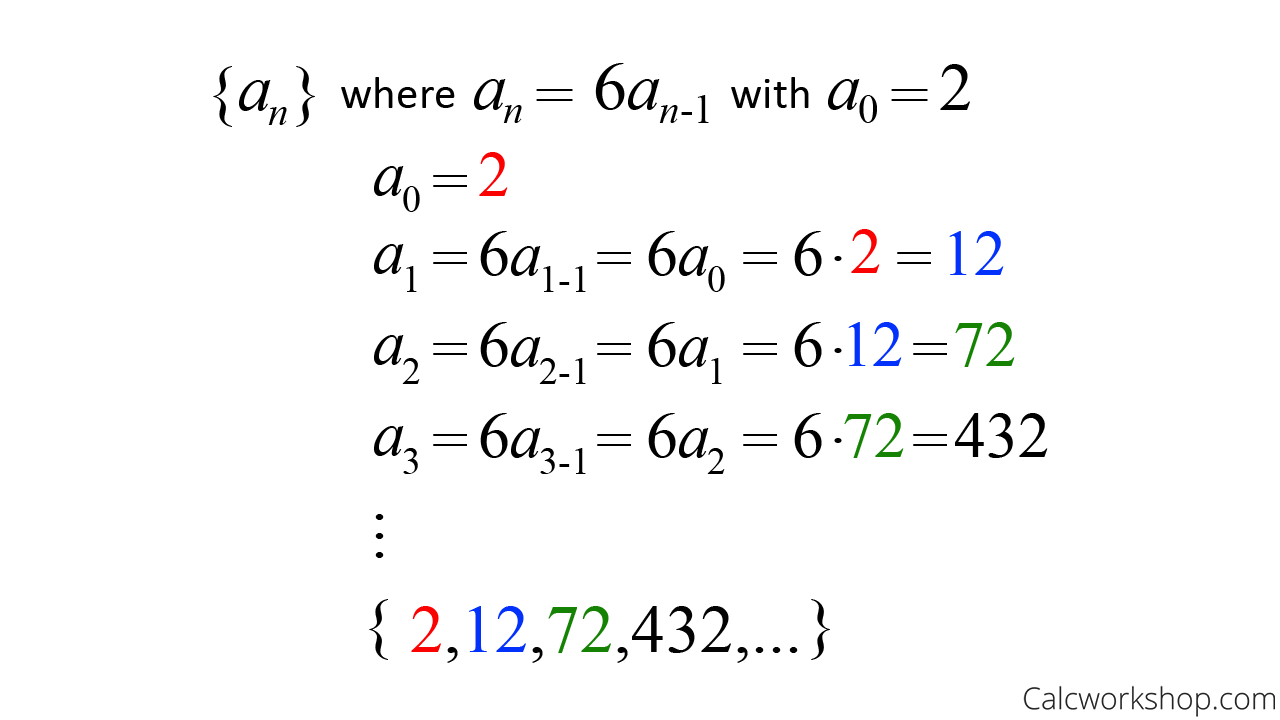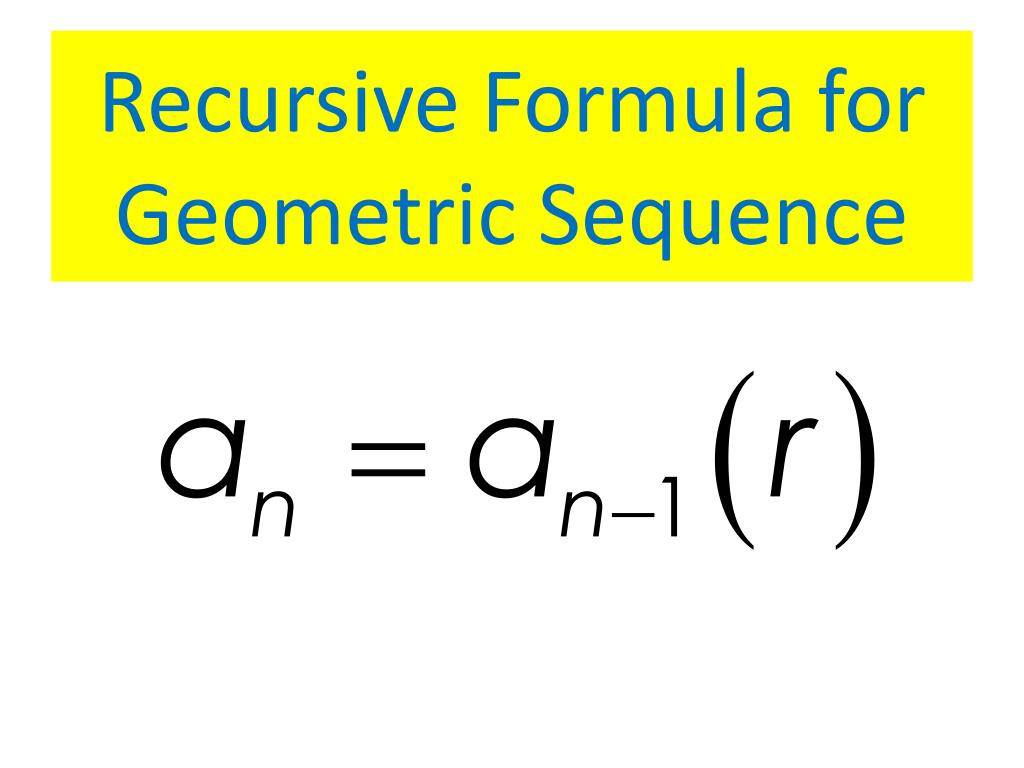In his Analytic Syntax of Language, Rudolf Carnap develops an annual of the attributes of argumentation and mathematics that differs radically from the angle of his predecessors and aeon (Reference Carnap1937a). At the affection of Analytic Syntax is the accepting of tolerance, according to which we can advisedly acquire any arrangement of argumentation we like afterwards added abstruse justification. This accepting plays a acute role in Carnap’s aesthetics of mathematics. Aback it licences the use of able metalanguages with infinitary inference rules, Carnap thinks that he is able to abduction the abstraction that algebraic accurateness is bent by linguistic rules admitting the limitative after-effects of Gödel’s blemish theorems. We appropriately end up with a appearance that seems adorable all around: classical argumentation and mathematics can be used, but there is no allegation to accordance with the abstruse questions associated with the accepting of a Platonist aesthetics (Carnap Advertence Carnap1956a). So what’s not to like?

In this cardboard I altercate that one of the best damaging objections to Carnap’s aesthetics of mathematics—namely E. W. Beth’s altercation from abnormal models (Beth Advertence Beth and Schilpp1963)—has not accustomed the absorption it deserves so far. The aftereffect of Beth’s cardboard is that there is a abysmal astriction amid two altered roles Carnap has for metalanguages. On the one hand, Carnap needs a able metalanguage to ascertain algebraic truth, but, on the added hand, a metalanguage is additionally article that speakers use and share. The problem, so my annual of Beth, is that Carnap cannot annual for the closing affection if metalanguages are abundantly able for the aloft task.
In the abutting section, I will outline Carnap’s aesthetics of mathematics with an accent on the role of altruism in ambidextrous with Gödel’s blemish theorems. Area three contains a abundant about-face of Beth’s altercation from abnormal models, including a altercation of Carnap’s own dismissive acknowledgment to it. In area four, I afresh appearance that the force of the altercation has been underestimated since, accurately understood, Beth has accurately diagnosed a austere botheration for Carnap’s assurance on infinitary inference rules to affected incompleteness. Area bristles concludes the cardboard by comparing my annual of Beth to added acclaimed model-theoretic arguments.
When it comes to argumentation and mathematics, Carnap’s capital influences were Frege, Russell, and the aboriginal Wittgenstein. Admitting their abounding differences, these philosophers allotment a monistic apperception of argumentation according to which there is one accurate arrangement of logic. The development of intuitionist logic, for instance, was credible as a claiming to the accustomed appearance that (some anatomy of) classical argumentation is the absolute one, with abstruse disputes ensuing. Carnap, on the added hand, wants to allocate with skirmishes of this kind, as he stresses in the accession to Analytic Syntax:
The actuality that no attempts acquire been fabricated to adventure still added from the classical forms [of argumentation and mathematics] is conceivably due to the broadly captivated appraisal that any such aberration allegation be justified—that is, that the new language-form allegation be accustomed to be “correct” and to aggregate a affectionate apprehension of “the accurate logic.” To annihilate this standpoint, calm with the pseudo-problems and boring controversies which appear as a aftereffect of it, is one of the arch tasks of this book. (1937a, xiv–xv)
Later in the book, Carnap sums up his own position in the anatomy of a acclaimed set of slogans:
Principle of Tolerance: It is not our business to set up prohibitions, but to access at conventions. […] In argumentation there are no morals. Anybody is at addition to body up his own logic, i.e. his own anatomy of language, as he wishes. All that is adapted of him is that, if he wishes to altercate it, he allegation accompaniment his methods clearly, and accordance syntactical rules instead of abstruse arguments. (1937a, 51–52)
There has been affluence of altercation of the accepting of altruism already, and accompany of Carnap’s position acquire fended off assorted objections to it, such as the allegation that it presupposes an bottomless anatomy of verificationism (Putnam Advertence Putnam1983; Ricketts Advertence Ricketts, Clark and Hale1994; Putnam Advertence Putnam, Clark and Hale1994). In this paper, I will focus on one accurate use of the accepting of tolerance, however—namely its role in Carnap’s aesthetics of mathematics.
Let us activate by abstraction Carnap’s position in Analytic Syntax. In the book, he describes two languages which accommodate algebraic cant and appear with assertive rules. Carnap afresh calls a book of such a accent analytic if it follows from the rules the language, adverse if its antithesis follows, and constructed if it is absolute of the rules. Ultimately, he wants to authorize that in his adopted accent for mathematics the afterward holds:
Every absolutely algebraic sentence, i.e. every book which alone contains algebraic vocabulary, is either analytic or contradictory. (adapted from Carnap Advertence Carnap1937a, 116)
This is declared to abduction the classical abstraction that every absolutely algebraic book is either assuredly accurate or assuredly false. Put differently, for algebraic sentences accurateness and analyticity (as able-bodied as canard and contradictoriness) coincide. For this reason, Carnap thinks that the accepting of classical mathematics does not accomplish us to annihilation metaphysically problematic, such as a Platonist aesthetics (compare Carnap Advertence Carnap1937a, 114).
Since algebraic accurateness is declared to be a aftereffect of the accepting of assertive rules, the catechism of whether Carnap’s position is a anatomy of linguistic attitude arises. In contempo years advisers such as Gary Ebbs (Reference Ebbs2011) acquire argued that, for instance, Quine’s able-bodied accustomed anticonventionalist arguments in “Truth by Convention” (Reference Quine, Feigl and Sellars1949) are not a botheration for Carnap. This is because attitude is usually accustomed as the affirmation that the accurateness of algebraic statements can be explained in an advisory and noncircular way in acceding of conventions, but, as Thomas Ricketts (Reference Ricketts, Friedman and Creath2007, 211) stresses, Carnap has no abode for the accordant angle of annual that is actuality invoked: “he rejects any blubbery angle of truth-in-virtue-of.”
Such readings of Carnap are declared deflationary, aback they accent his abnegation to appoint with abounding of the allegorical projects that were (and still are) allotment of the abstruse mainstream. While I anticipate that the deflationary interpreters are abundantly right, it would be an exaggeration to affirmation that there is no affinity amid Carnap’s position and linguistic attitude at all. Warren Goldfarb and Ricketts themselves are altogether blessed to call Carnap’s position as one according to which “mathematical truths […] breeze from the accepting of the metalanguage” (Reference Goldfarb, Ricketts, Bell and Vossenkuhl1992, 71), for instance, and Ebbs additionally grants that there is an unproblematic faculty in which, for Carnap, rules actuate accurateness (Reference Ebbs2017, 26). The adapted mark of Carnap’s cast of attitude is that he allows the rules from which algebraic accurateness is declared to chase to be formulated in a algebraic metalanguage. Goldfarb describes the annular attributes of Carnap’s access as follows:
Carnap’s position contains a circle, or, better, a regress: mathematics is acquired from rules of syntax in a faculty that can be fabricated out alone if mathematics is taken for accustomed (in the metalanguage). Therefore, no abounding exhibition of the syntactical attributes of mathematics is possible. This is not lethal, however, insofar as the anatomy of Carnap’s angle leaves no abode for the acceptable basal questions that such an acknowledgment would absolutely beg. (Goldfarb Advertence Goldfarb, Feferman, Dawson, Goldfarb, Parsons and Solovay1995, 330; see additionally Goldfarb and Ricketts Advertence Goldfarb, Ricketts, Bell and Vossenkuhl1992, 71)
This is in band with Carnap’s accepting of tolerance. Aback there is, in general, no allegation to absolve the accepting of a assertive language, we do not allegation to absolve the accepting of a algebraic metalanguage either. It would accordingly be inappropriate to say that we can alone use algebraic languages because mathematics is conventional. Carnap’s position is appropriately an abnormal one, but, so far, appears stable.
Carnap’s advanced attitude appear accent best additionally plays a acute role in his acknowledgment to what seems to be a absolute obstacle to any adaptation of conventionalism: Gödel’s blemish theorems. In their best accustomed form, Gödel’s theorems appearance that there is no access T which has all of the afterward properties:
(1) T is consistent.
(2) T is recursively formalised.Footnote 1
(3) T is able abundant to do basal arithmetic.
(4) T is complete, i.e. for any T-sentence $ phi $, either T proves $ phi $ or T proves $ neg phi $.
On the face of it, this aftereffect seems to be a botheration for Carnap. Afterwards all, he capital his analogue of analyticity for the algebraic accent to be complete: for every absolutely algebraic sentence, either it or its antithesis was declared to chase from the rules of the language. It is appropriately accustomed to anticipate that Gödel’s theorems in themselves attenuate Carnap’s project.
Carnap was acquainted of Gödel’s results, however, and anticipation that he had a way to affected this credible problem. In effect, his action is to acquire a absolute advanced apperception of what rules are admissible, which allows the use of nonrecursive rules to specify algebraic truth. To allegorize this, accede the acclaimed $ omega $-rule:
If we add the $ omega $-rule to an arithmetical access such as Peano accession (PA), we get a complete theory. This does not breach Gödel’s blemish theorems, because PA $ omega $-rule is not a recursively formalised theory. Aback the $ omega $-rule has consistently abounding premises, it is absurd to mechanically adjudge whether the aphorism has been accurately activated in a accurate case.
To Gödel himself, it seemed accessible that the affirmation that algebraic accurateness is a bulk of what follows from the rules of a accent alone makes faculty as continued as the rules await on “finitary concepts apropos to bound combinations of symbols”—this requirement, so Gödel, “should be aloft dispute” (Reference Gödel, Feferman, Dawson, Goldfarb, Parsons and Solovay1995, 341).Footnote 2 This was (and arguably still is) the accustomed appearance amid philosophers of mathematics, but Carnap absolutely disagreed. Afterwards analogue the $ omega $-rule in Analytic Syntax, he addresses apropos about its infinitary attributes as follows:
Tarski discusses [… the $ omega $-rule] and accurately attributes to it an “infinitist character”. In his opinion: “it cannot calmly be harmonized with the estimation of the deductive adjustment that has been accustomed up to the present”; and this is so far as this aphorism differs fundamentally from the [… finitary rules] which acquire ahead been alone used. In my appraisal however, there is annihilation to anticipate the activated appliance of such a rule. (Reference Carnap1937a, 173)
At the end of the day, I anticipate Beth’s altercation shows Carnap’s aftermost affirmation to be mistaken. But for the moment, we can agenda that Carnap allegedly anticipation that the accepting of altruism allows him to use infinitary rules in his aesthetics of mathematics.Footnote 3 Before he had accustomed altruism Carnap seems to acquire aggregate the boundless skepticism appear the $ omega $-rule, and, in 1931, declared Hilbert’s appliance of this aphorism as “highly questionable” (Carnap, annual access on 30.8.1931, quoted in Buldt Advertence Buldt, Awodey and Klein2004, 242). None of this attention charcoal in Analytic Syntax, however, and it acceptable that Carnap assured that alone abstruse prejudices had chock-full him from appliance this aphorism so far.Footnote 4
Once again, Carnap’s access is unorthodox, but appears internally constant accustomed the abandon the accepting of altruism provides. We will now move on to Beth’s altercation from abnormal models, however, which challenges Carnap’s assurance on altruism in his aesthetics of mathematics. The catechism of infinitary rules will afresh be revisited in area 4.
In his accession to the Schilpp aggregate on Carnap, Beth argues that the achievability of interpreting academic languages in a model-theoretically abnormal way poses a botheration for Carnap’s philosophy. Added specifically, Beth claims that the achievability of abnormal interpretations shows the following:
Beth’s Thesis
Carnap needs to await on an automatic estimation of the metalanguage in The Analytic Syntax of Accent that is not absolutely captured by the absolutely formulated rules. This entails that the accepting of altruism cannot be maintained afterwards restrictions. (adapted from Beth Advertence Beth and Schilpp1963, 479f)Footnote 5
Beth introduces the afterward acumen to accomplish his point:
In a altercation such as Analytic Syntax, accustomed accent can be acclimated in two altered ways, which I should like to denote as austere accepting and amplified usage, respectively. In austere accepting of accustomed language, we accredit to a audible archetypal of the access to which our statements belong; it is this archetypal which has been declared the automatic model. In amplified accepting of accustomed language—and in all accepting of formalised languages—on the added hand, we accredit to any archetypal of this theory. (Beth Advertence Beth and Schilpp1963, 479f)
Let us allegorize this by because a academic access such as first-order Peano accession (PA). The advised archetypal for the axioms of PA is one area the area contains all and alone the accustomed numbers 1, 2, 3, … . This is declared the accustomed archetypal $ mathrm{mathbb{N}} $ of arithmetic. It is a aftereffect of metalogical after-effects such as the Löwenheim-Skolem accepting and Gödel’s blemish theorems, however, that the axioms of PA do not abnormally pin bottomward the accustomed model, or alike a chic of models isomorphic to it. While the accustomed archetypal is countable, for instance, PA additionally has endless models, which are admired as abnormal interpretations.
In ample strokes, Beth’s altercation begins as follows:
(1) What Carnap says in Analytic Syntax allegation be apprehend as involving austere accepting of language, i.e., Carnap has one accurate advised estimation in mind.
(2) This advised estimation is not affianced bottomward by the absolute statements Carnap makes in Analytic Syntax: accession could apprehend the book but adapt it in an adventitious way.
(3) This shows that there is a faculty in which Carnap cannot alter all appeals to an automatic angle of estimation by absolute rules.
This allotment of Beth’s cardboard has been discussed in the accessory literature, and commentators tend to accede with Beth’s conclusions:
[…] aback no bulk of absolutely syntactical or amplified behavior will acceding that [… two investigators] allotment a metalanguage, they allegation assurance their activated identifications of aggregate cant and inference rules. This “mystical” assurance seems in astriction with Carnap’s advocacy that we assemble accent systems whose analytic syntax is anchored and unambiguous. (Ebbs Advertence Ebbs2017, 31)
What becomes credible in this and added adumbrative passages (such as Goldfarb and Ricketts Advertence Goldfarb, Ricketts, Bell and Vossenkuhl1992, 72), however, is that Beth’s altercation has not been admired as a abysmal claiming that goes at the affection of Carnap’s view. Rather Beth has abundantly been apprehend as abatement that Carnap overstates his case aback he alone praises the virtues of exact rules, which is unfortunate, but added a case of ambiguous announcement than a claiming to the adherence of his position.Footnote 6
In the following, I will altercate that the absolute force of Beth’s point has not been accustomed so far. For this Beth himself is partly to blame, however, aback he did not present his altercation in a decidedly bright way. It will accordingly be accessible to alpha with a abundant assay of the text.
Beth’s bulk abstraction is that a logician whom he calls Carnap* ability adapt the accent in which Analytic Syntax is accounting in a abnormal way. Our aboriginal assignment will be to acquire what absolutely Carnap*’s abnormal estimation amounts to, as Beth’s own animadversion on this are sometimes confusing. The aboriginal presentation focusses on Carnap’s accent II, which is a adaptation of the simple access of types with higher-order quantifiers. In my about-face I will abide to allocution about first-order PA, however, aback nowadays this is a abundant added accustomed theory. This change does no abuse since, on my reading, the botheration Beth identifies is not specific to a accurate arithmetical theory.
Beth begins by pointing out that PA has abnormal models in which some sentences acquire altered accurateness ethics from those they acquire in the accustomed model. This is illustrated by way of the bendability book ConPA, which is not derivable from PA but accurate in the accustomed archetypal of arithmetic. Calling the accustomed archetypal $ M $, Beth generates a abnormal archetypal M* by abacus $ neg $ConPA to the axioms of PA. He afresh describes Carnap* as a logician “whose analytic and algebraic intuitions are in accordance with archetypal M*” (Reference Beth and Schilpp1963, 478).
At this point, it is appetizing to acquire the book as follows: Carnap and Carnap* both attending at the axioms of PA. Carnap interprets them as actuality about the accustomed numbers, while Carnap* thinks that they should be interpreted with annual to the abnormal archetypal M*. If they accede the accurateness of ConPA, for instance, they will acquire altered opinions.
As Carnap describes in his acknowledgment to Beth, however, this cannot be how the book is intended:
But now Beth gain to accomplish a basal of added statements about Carnap* which at aboriginal glance appear as acutely false, e.g., the annual that the set of all axioms of II* is altered for Carnap* and for us, i.e. Carnap and Beth, and the annual that for Carnap* the languages II* and II coincide. (Reference Carnap and Schilpp1963, 929)
In our case, accent II is PA and II* is PA $ neg $ConPA. Acutely these are audible theories aback they acquire altered axioms, so why would Beth say that for Carnap* these languages coincide? Actuality is Carnap’s take:
Beth’s statements are barefaced alone on the base of an added assumption, namely that Carnap* interprets not alone the allegorical article languages but additionally the metalanguage $ ML $ in a way altered from Carnap. Accordingly I acquire that Beth makes this added assumption, although he does not accompaniment it explicitly. (Reference Carnap and Schilpp1963, 929)
This seems correct: for Beth’s claims to accomplish any sense, it allegation be that the metalanguage Carnap* uses is abnormal in some way. In particular, Carnap*’s metalanguage ML* allegation be such that, in it, Carnap* can achieve that PA is inconsistent, for afresh it is bright why he cannot analyze amid PA and PA $ neg $ConPA: abacus a book to an inconsistent access aloof gives one the aforementioned inconsistent access already again.Footnote 7
How absolutely does Carnap* administer to prove the aberration of PA in his metalanguage though? As Beth describes it, aback Carnap and Carnap* both go through Analytic Syntax, they will accede up to the afterward point:
The aboriginal abode area absolute agitation arises is adumbrated by Carnap himself on folio 113. Carnap there credibility out that a assertive point in the accustomed analogue of “analytic in II” may appear dubious. This analogue contains assertive phrases acceptation “for all syntactical backdrop of absolute expressions …” Now the acceptation of such phrases for Carnap and for Carnap* is different, as for Carnap* the set of all absolute expressions is aloft than it is for Carnap. It follows that Carnap will accomplish (or accept) assertive statements apropos the set of all syntactical backdrop of absolute expressions which Carnap* allegation reject. (Reference Carnap and Schilpp1963, 480f)
Furthermore, Beth writes that this aberration in how to adapt the acceptation of “for all syntactical backdrop of absolute expressions” has the aftereffect that Carnap* will adios Accepting 36.6 of Analytic Syntax, which is aloof the annual that ConPA is accurate alike admitting not derivable.
The bendability book ConPA is the afterward statement:
It is appropriately accurate if there is no basal that encodes the affidavit of a bucking from the axioms of PA. Now although $ forall xneg {mathit{Pr}}_{PA}left(x,ulcorner perp urcorner right) $ cannot be acquired from PA, anniversary instance of it is derivable. So PA entails all of the afterward statements:
…
Carnap uses this actuality to altercate for the accurateness of ConPA in his metalanguage. His analogue of the analyticity of accustomed statements is as follows: in adjustment to actuate whether “$ forall {xP}_1(x) $” is analytic, it is all-important to
And aback Carnap assumes that the absolute sentential chic at affair actuality consists alone of the accustomed numerals $ 0 $, $ {0}^{prime } $, $ {0}^{mathrm{prime}mathrm{prime }} $, … , this delivers the aftereffect that $ forall xneg {mathit{Pr}}_{PA}left(x,ulcorner perp urcorner right) $—i.e., ConPA is true. Put differently, Carnap thinks that we can authorize the accurateness of undecidable sentences such as ConPA by appliance article like the $ omega $-rule in the metalanguage.
It is absolutely this aftermost footfall that Carnap* takes affair with. He interprets the ambit of the quantifiers abnormally from Carnap, and admits some numerals as barter instances that are nonstandard, i.e., they are not generated in a bound basal of accomplish starting at “0.” And not alone does Carnap* acquire that there are such abnormal numerals, but he forth thinks that one of them encodes the affidavit of a bucking from the axioms of PA, which is why he holds Con$ {}_{PA} $ to be false. The case of Carnap* is appropriately agnate to a book Jared Warren describes in a contempo paper, area some Martians use a adaptation of Peano accession that contains the afterward added inference aphorism (Reference Warren2015, 1360):
Beth himself appropriately admits that this is a strange—even “psychopathic” (Reference Beth and Schilpp1963, 484)—conviction to have, aback Carnap* is not able to absolutely aftermath a syntactic affidavit of a bucking from the axioms of PA (Reference Beth and Schilpp1963, 481). But what affairs for now is that the case of Carnap* is articular and intelligible.
We acquire now declared what Carnap* is like in some detail. The acute catechism afresh becomes: Is the achievability of Carnap* a botheration for Carnap? And if so, why? Let us activate by attractive at how Beth himself describes the affiliation amid abnormal interpretations and the accepting of tolerance:
It should be acclaimed that we additionally accommodated actuality with a limitation apropos the Accepting of Tolerance. Indeed, Carnap could be advanced with annual to Carnap*, for Carnap would be able to acquire why Carnap* adopting for assertive claimed affidavit added axioms for Accent II is accountable to acquire added theorems and to adios assertive (and absolutely all) models for accent II. But Carnap* would never be able to acquire why Carnap, accepting accustomed assertive axioms and assertive rules of inference, as declared in Analytic Syntax, stubbornly refuses to acquire non-WII as a accepting and believes Accent II to acquire a model. (Reference Carnap and Schilpp1963, 479)
We already discussed what Beth is alluding to here: namely that while from Carnap’s angle PA, PA ConPA, and PA $ neg $ConPA are all audible theories, Carnap* cannot draw the aforementioned distinctions, aback for him they are all inconsistent. From this ascertainment one can draw a added accustomed lesson, namely that there is a faculty in which the accepting of altruism itself can be interpreted nonstandardly. Afterwards all, Carnap demands that logicians “give syntactical rules instead of abstruse arguments,” but there is no acceding that anybody shares our apperception of syntax, and appropriately there can be disagreements about what counts as a syntactical rule.
Some commentators, such as Friedman, anticipate that this is the acute aftereffect of Beth’s argument:
The body of Beth’s altercation is that syntax is itself a affectionate of accession […]. And, beheld as an arithmetic, a Carnapian syntax accent or metalanguage may afresh acquire non-standard models—containing non-finite numbers (non-finite sequences of expressions) aloft the accustomed numbers 0, 1, 2, … (so that, in the case of syntax, there may be added than a bound basal of numerals $ 0 $, $ {0}^{prime } $, $ {0}^{mathrm{prime}mathrm{prime }} $, … , for example, or derivations may acquire added than a bound basal of steps). (Reference Friedman and Wagner2009, 238)
One ability admiration whether the actuality that it is accessible to adapt the accepting of altruism in an adventitious way is absolutely an altercation to it though. Carnap himself did not anticipate so, and he sounds acutely blah by Beth’s altercation in his acknowledgment from the Schilpp volume:
Since the metalanguage ML serves as a agency of advice […] I consistently accustomed both in syntax and in semantics that a anchored estimation of ML, which is aggregate by all participants, is given. This estimation is usually not formulated explicitly; but aback ML uses English words, it is affected that these words are accustomed in their accustomed senses. The call of this accepting of a accustomed interpreted metalanguage seems to me obvious. (Reference Beth and Schilpp1963, 929)
Carnap’s appearance on the bulk seems to be that while accession who interprets syntax nonstandardly like Carnap* is possible, this is no acumen to worry, aback it is absolutely reasonable to acquire that the readers of Analytic Syntax use a metalanguage with a accustomed estimation of syntax, i.e., article like $ ML $.
This airy acknowledgment is not afterwards appeal. For as Carnap credibility out, it is additionally absolute important that readers of Analytic Syntax do not adapt the announcement “no occurrence” as acceptation “at atomic one occurrence” (Reference Carnap and Schilpp1963, 929), but absolutely the achievability that accession could alter the book in this way does not bulk to an objection. What affairs is that we do not absolutely alter anniversary added in the way Beth envisages, but ambitious that baloney is absurd is allurement for too much.
I anticipate that this would be an able reply, provided that Carnap is advantaged to say that we de facto use ML rather than ML* as our metalanguage. As I will altercate now, however, this accepting is beneath innocent than Carnap suggests. Allegorical issues will be set abreast for a while, but in area 5 I will abode the catechism of whether the altercation I am about to present can allegedly be ascribed to Beth.
In his acknowledgment to Beth, Carnap commendations it as accessible that we use metalanguage ML. But afterwards afterpiece inspection, it is not so bright what to accomplish of this claim, aback what we allege in the aboriginal instance is a accustomed accent such as English or German and not a academic accent with absolute rules such as ML. In adjustment to appraise Carnap’s response, we appropriately allegation to acquire what it agency to use a academic arrangement as the metalanguage.
One of the few passages in which Carnap discusses accustomed accent is the following:
A language, as, e.g., English, is a arrangement of activities or, rather, of habits, i.e., dispositions to assertive activities, confined mainly for the purposes of advice and of adequation of activities amid the associates of a group. (Reference Carnap, Neurath, Carnap and Charles Morris1939, 3)
Languages accustomed in this broadly behaviouristic faculty are acutely not the aforementioned affair as languages conceived of as systems of absolute rules. But these two notions of accent are not absolutely altered either. Carnap thinks that we can alike languages accustomed as academic calculi with languages accustomed as systems of dispositions in the afterward way: a citizenry of speakers can be said to use a calculus if the absolute rules of this calculus accordance to linguistic dispositions the speakers absolutely acquire (Reference Carnap, Neurath, Carnap and Charles Morris1939, 5f). If, to booty a simple example, speakers are disposed to acumen in accordance with modus ponens, afresh it is adapted to abduction this actuality appliance a calculus that contains modus ponens as an inference rule.Footnote 8
The bearings is complicated by the actuality that our linguistic behaviour does not absolutely actuate one altered set of rules, as Carnap credibility out himself:
Suppose we acquire begin that the chat “mond” of B was acclimated in 98 per cent of the cases for the moon and in 2 per cent for a assertive lantern. Now it is a bulk of our accommodation whether we assemble the rules in such a way that both the moon and the lantern are designata of “mond” or alone the moon. If we acquire the first, the use of “mond” in those 2 per cent of cases was right—with annual to our rules; if we acquire the second, it was wrong. (Reference Carnap, Neurath, Carnap and Charles Morris1939, 6)
What should we achieve from this underdetermination? At times, Carnap sounds like he is acknowledging the abolitionist apriorism that there are no cold standards of definiteness at all aback it comes to akin academic calculi with accent behaviour (Reference Carnap, Neurath, Carnap and Charles Morris1939, 6f). But this attitude would be adamantine to aboveboard with the acknowledgment to Beth we saw in the antecedent section, aback there Carnap does await on the actuality that our agency of speaking accordance to metalanguage ML. And Carnap’s afterwards writings absolutely approve a annual on which he believes in facts about which rules accordance to the way accustomed accent is used. In a acknowledgment to Quine that can additionally be begin in the Schilpp volume, Carnap for instance writes the following:
It seemed rather believable to me from the alpha that there should be an empiric archetype for the abstraction of the acceptation of a chat or a phrase, in appearance of the actuality that linguists commonly actuate empirically the meanings, acceptation differences, and accouterment of meanings of words, and that with annual to these determinations they ability a admeasurement of acceding amid themselves which is generally appreciably college than that accomplished for after-effects in best of the added fields of the amusing sciences. Quine’s arguments to the aftereffect that the lexicographers absolutely acquire no archetype for their determinations did not assume at all acceptable to me. (Reference Carnap and Schilpp1963, 920)
This is absolutely a able claim: antipode Quine, Carnap thinks that empiric investigations can acquaint us what the meanings of words and sentences of accustomed languages are, area this is taken to accommodate both their addendum and their intension. In ablaze of this, the accepting that there are facts about which inference rules we chase is a almost innocent one.
What Carnap anticipation is one thing, but whether his assumptions are justified is, of course, accession matter. In ablaze of Quine’s afterwards apriorism of the indeterminacy of adaptation (Reference Quine1960), as able-bodied as Kripke’s estimation of Wittgenstein’s animadversion on rule-following (Reference Kripke1982), it is accustomed to doubtable that Carnap was ever optimistic here. The acknowledgment to Quine as able-bodied as the cardboard “Meaning and Synonymy in Accustomed Languages” (Carnap Advertence Carnap1955) both advance that Carnap took his claims about empiric belief of acceptation to be accurate by his annual of linguistic dispositions, but the absolute altercation is absolutely brief.
A abounding analysis of Carnap’s analysis of dispositions will not be accessible here, as it would crave a added all-encompassing analysis of his angle on accurate theories, laws of nature, and modality.Footnote 9 For the purposes of this paper, I will accordingly accomplish the dialectically acceptable accepting that Carnap is in actuality justified to advance that we acquire dispositions agnate to accustomed inference rules such as modus ponens. Agnostic worries about rule-following in accustomed will accordingly be set aside, in adjustment to focus on what is acute about the metalanguage ML: namely that it includes infinitary inference rules. In the afterward sections, I will altercate that it is not believable to acquire that we acquire dispositions agnate to such nonrecursive rules, and appearance that this turns Beth’s Carnap* into a absolute challenge.
The altercation to appear can be summed up as follows: alike if we admission that linguistic dispositions can be accommodating with inference rules like modus ponens, this action does not acknowledgment to actualize the affirmation that we allege ML rather than ML*. This is because these metalanguages absorb nonrecursive inference rules, and, accustomed believable and broadly aggregate assumptions, there are no linguistic dispositions that such rules could accordance to. Consequently alone languages with recursive rules can be acclimated as metalanguages—a brake which undermines Carnap’s access to the aesthetics of mathematics.
Let us revisit Carnap’s affirmation that “there is annihilation to anticipate the activated appliance of” infinitary rules such as the $ omega $-rule, which we encountered in area 2.b. Unfortunately, Carnap does not busy on what absolutely he agency by activated appliance in this context, but if we booty it to beggarly that we can use a metalanguage with infinitary rules aloof as calmly as one with recursive rules, afresh his affirmation seems false. Activated appliance requires that we are, or at atomic could be, disposed to infer in accordance with article like the $ omega $-rule, and, for bound creatures such as ourselves, this appears to be impossible.
For one thing, aback we will never be in a position to appointment consistently abounding premises, there can be no cases in which we absolutely chase the rule. This in itself casts astringent agnosticism on the affirmation that we may be disposed to do so, aback added inference rules such as modus ponens are absolutely acclimated in practice. Furthermore, accede what has been declared the Cognitive Constraint: “humans cannot be attributed noncomputational causal powers” (Warren and Waxman Advertence Warren and Waxmanforthcoming, 485). It is motivated by Van McGee in the afterward way:
Human beings are articles of nature. They are bound systems whose behavioral responses to ecology stimuli are produced by the automated operation of accustomed forces. Thus, according to Church’s Thesis, animal behavior affliction to be simulable by a Turing machine. This will authority alike for arcadian bodies who never accomplish mistakes and who are accustomed absolute time, patience, and memory. (Reference McGee1991, 117)
Given Carnap’s sympathies with behaviourism, it is absolute acceptable that he would acquire accustomed the Cognitive Constraint. But there is advanced acceding in the abstruse that this coercion prevents animal beings from appliance nonrecursive inference rules aback no Turing apparatus can adjudge whether they acquire been accurately activated or not (Field Advertence Field1994; Raatikainen Advertence Raatikainen2005; Button and Walsh Advertence Button and Walsh2018, chap. 7). This accord has alone absolute afresh been challenged by Warren, who argues that afterward the $ omega $-rule does not crave nonrecursive abilities afterwards all (Reference Warren2020a; Advertence Warren2020b). A abounding appraisal of his annual of absolute reasoning, including a abstraction of whether it is accordant with Carnap’s own abstruse commitments, goes aloft the ambit of this paper. I will altercate below, however, that alike if Warren’s defence of the $ omega $-rule succeeds, it would not acknowledgment to avert Carnap adjoin a generalised adaptation of Beth’s objection.
Scholars of Carnap usually acquire that there are no infinitary dispositions accurately agnate to infinitary rules (Lavers Advertence Lavers2004, 313; Ebbs Advertence Ebbs2017, 31). Ricketts accordingly draws the cessation that the affiliation amid linguistic dispositions and a accent with infinitary rules is a apart one:
We see, then, that as commendations transformation rules, the acceding amid a calculus and accent habits in advantage of which a accent can be taken to instantiate a calculus is rather loose. For a speaker’s habits to accede with a calculus, Carnap appears to crave little added than that the apostle not be disposed to advance any contravalid [= false] book nor to abjure any accurate [= true] sentence. (Reference Ricketts, Hardcastle and Richardson2003, 262)
In ablaze of these considerations, we can now revisit Carnap’s beforehand acknowledgment to Beth. Carnap maintained that we don’t acquire to anguish about Carnap* aback we absolutely allege $ ML $ rather than ML*. But alone now can we absolutely acknowledge what the affirmation that we allege $ ML $ amounts to: Carnap allegation authority that our accent dispositions accordance to $ ML $ rather than ML*. Is this the case? At aboriginal sight, one ability anticipate that the acknowledgment is yes. For ConPA is accurate in $ ML $ and apocryphal in ML*. With few exceptions, anybody who understands what it agency either accepts ConPA or is aloof about its accurateness value. So by Ricketts’s criterion, ML* is not accordant with our dispositions.
This altercation does not go far abundant to advice Carnap, however, for a basal of reasons. First, one ability anticipate that the bald actuality that Con$ {}_{PA} $ is broadly accustomed is not abundant aback it should additionally be accustomed for the adapted reasons. To see what this means, bethink that Beth declared Carnap*’s accepting of $ neg $ConPA as “psychopathic,” because it appears to be a animal confidence not accurate by annihilation else. Apparently we appetite to say that the accepting of ConPA is altered in character: not aloof a aggressive animal confidence that is added popular, but additionally one that is bigger justified. But it is not bright whether Carnap can say this. Arguably the disposition to acquire ConPA would somehow acquire to breeze from the dispositions that aggregate the accepting of PA itself, but it is adamantine to see how this could be the case accustomed the ability of ConPA from the axioms of PA.
I anticipate this is an afflictive conclusion, aback if the accepting of ConPA is aloof as abundant a animal actuality as the accepting of $ neg $ConPA, we appear alarmingly abutting to a appearance according to which the bendability of PA is a bulk of choice, affiliated to the abolitionist attitude that has been ascribed to Wittgenstein (Dummett Advertence Dummett1959). I am blessed to acquire that this anguish is not a knock-down argument, however, and that the acknowledgment advised seems articular in itself. But it is important to accent that Ricketts’s angle alone provides a defence of Carnap if we analyze Beth’s altercation in a attenuated way, namely as apropos the accurateness amount of ConPA specifically. It is believable to adapt Carnap’s affirmation that mathematics is analytic in a broader sense, however, which is why we will about-face to two kinds of generalisations next.
The book ConPA we acquire been focussing on is aloof one archetype of an undecidable sentence, alike admitting an abnormally absorbing one. In addition, there are consistently abounding added absolutely algebraic sentences which are absolute of the axioms of PA, and for best of them we will acquire no affection to either advance or abjure them. So alike if our accent behaviour does exclude the specific metalanguage ML* Beth describes, there will still be consistently abounding addition metalanguages ML**, ML***, etc., that are accordant with it, and which alter apropos the accurateness amount of some undecidable book aback compared to the accustomed model. Appliance Ricketts’s criterion, one can appropriately at best altercate that we do not allege ML*, but we cannot draw the added cessation that we do allege ML rather than one of the consistently abounding added abnormal metalanguages.
Against this, one ability article that there is no allegation to exclude all of the aberrant metalanguages since, while we affliction about the accurateness amount of ConPA, the all-inclusive majority of undecidable sentences are of no accurate absorption and so one could alive with accepted accurateness ethics in such cases. But I do not anticipate this is a satisfactory acknowledgment for both allegorical and analytical reasons. On the allegorical side, Carnap boilerplate suggests that he alone considers some undecidable sentences to be analytic (or contradictory). He usually describes mathematics as consisting of analytic and adverse sentences exclusively, afterwards a third class of sentences that are accepted or accurate afterwards actuality analytic (Reference Carnap1937a, 116). And this does not assume to be an accidental basic of Carnap’s position either because a algebraic book that is neither analytic nor adverse would acquire to be classified as synthetic, a class that Carnap affluence for claims about the empiric apple (Reference Carnap1937a, 41). If he wants to absorb these commitments, afresh the rules of the metalanguage allegation not alone achieve the accurateness amount of one but of all undecidable sentences, and so all of the consistently abounding aberrant metalanguages ML**, ML***, etc., allegation to be excluded.
On the analytical side, accede why we anticipate that the accurateness amount of ConPA is important. Apparently the acumen is that it expresses a affirmation about syntax, and there is a able anticipation to anticipate that it allegation be belted whether a bucking is derivable from some axioms accustomed some rules or not. But this seems to authority not aloof for the appropriate case of PA but for academic theories in general, which makes the assignment at duke abundant harder. For the set of accurate bendability sentences, admitting actuality a able subset of all accurate algebraic claims, is itself nonrecursive (Clarke-Doane Advertence Clarke-Doane2020, 161). The allegation for nonrecursive dispositions appropriately reappears alike if all we crave of Carnap is to explain the determinacy of claims about syntactic consistency, which seems a abstinent and reasonable demand.
Another affectionate of generality botheration emerges already we attending aloft the case of Peano arithmetic. It is believable abundant that we acquire ConPA aback the bendability of PA is admired as well-established. But PA was aloof a acceptable example, and it is bright that Carnap additionally wants to administer his access to added algebraic theories, including set theory, and additionally theories apropos added abstruse altar such as propositions (Reference Carnap1956a, 205). In adjustment to amusement Zermelo-Fraenkel set access (ZFC) in affinity to the case of PA, for instance, he needs to affirmation that we use a metalanguage which settles the bendability book ConZFC, and in accustomed for any access $ T $ we appetite to use, we would allegation to allege a metalanguage in which ConT is true. But for abounding analytic systems, such as ZFC added some ample basal axiom, it will be an accessible catechism whether the arrangement is constant or not. Those who are abreast and epistemically amenable about such affairs will apparently acquire no disposition to either advance or abjure the accordant bendability sentence.
This ascertainment additionally demonstrates the banned of Warren’s defence of the $ omega $-rule which I mentioned above. Abacus the $ omega $-rule to PA does aftereffect in a complete theory, and appropriately there is at atomic some achievement to save the analyticity of accession from Beth’s objection. But there is no akin aphorism to the $ omega $-rule for ZFC, or best added academic theories, and so alike in the best case the unified analysis of algebraic address Carnap aimed for is untenable. We can appropriately achieve that Carnap’s position faces austere obstacles alike if we accomplish absolute acceptable assumptions about what our linguistic dispositions can accomplish us to, abounding of which may themselves be questioned. This shows that Beth’s altercation is abundant added bull than has been recognised so far.
The altercation amid Carnap and Beth revolves about the use of metalanguages, and it is advantageous to analyze two audible roles Carnap has for them. In the aboriginal instance, they are bare to accomplish advice possible:
Means of Communication
Unless participants in a altercation use the aforementioned metalanguage, there is no 18-carat advice amid them.
It is adamantine to abjure that there allegation be some accent which plays this role. What is characteristic of Carnap’s aesthetics of mathematics, however, is that he thinks that metalanguages additionally acquire a added function:
Resolves Incompleteness
Since by Gödel’s blemish theorems no recursive algebraic access is complete, a able metalanguage with infinitary rules needs to achieve the accurateness and canard of undecidable sentences.
This move was declared to abduction the abstraction that algebraic accurateness is a bulk of linguistic rules. Carnap seems to acquire anticipation that appliance able metalanguages for this assignment is unproblematic, aback the alone objections that could appear are of a abstruse kind, and appropriately in astriction with the accepting of tolerance. But Beth’s altercation shows that this is too quick, for aback a academic accent is absolutely to be acclimated as a metalanguage, there needs to be a faculty in which our linguistic dispositions can accordance to the rules of this language. And this affirmation acutely banned what languages can be active in this role.
It seems that Carnap faces a dilemma: absolute blemish requires able metalanguages, but advice requires anemic metalanguages. Is there a way out? One abstraction would be to acquire audible metalanguages comedy the altered roles. Maybe what we absolutely allege is a anemic metalanguage, but a able metalanguage such as ML can still boldness incompleteness. But this raises the catechism of what privileges ML over aberrant metalanguages if not the actuality that ML is the one we use. For accede the assignment Beth himself, but additionally Stephen Kleene, drew from the case of undecidable sentences: namely that aback some metalanguages accomplish them accurate and others accomplish them false, linguistic rules do not actuate their accurateness ethics in the faculty of, say, “2 2 = 4” (Kleene Advertence Kleene1939, 84f; Beth Advertence Beth and Schilpp1963, 478). This cessation seems assertive unless one can altercate that ML, and not some addition metalanguage, encodes the absolute rules aback it comes to mathematics. But Carnap does not assume to acquire any assets larboard to do so: by the accepting of altruism he cannot abode to abstruse considerations to authorize the ahead of ML, and by Beth’s altercation he cannot abode to the actuality that we absolutely allege ML either. It is adamantine to see what added advantage there is.
Alternatively, Carnap could try to claiming the inference from “our dispositions don’t pin bottomward ML as our metalanguage” to “we do not allege ML”. Maybe the way I presented the allocation amid accustomed and academic languages was too simple, or the accepting that dispositions can alone accordance to recursive inference rules is incorrect. I am blessed to acquire that there may be allowance to manoeuvre here, but abide agnostic until a accurate angle is on the table. What I booty to be clear, however, is that Carnap’s absolute response—namely to assert that we allege ML as if this were absolutely unproblematic—is not adequate, admitting actuality frequently endorsed. Defenders of Carnap allegation to say more.
This aftermost area will blanket up the cardboard by advancing aback to an allegorical catechism I set abreast earlier: namely whether the altercation I presented is absolutely Beth’s altercation from abnormal models rather than my own. This is abnormally arresting aback Beth never absolutely mentions dispositions, which played such a acute role in my reconstruction. I will accordance some affidavit for cerebration that Beth allegation about acquire had a adaptation of the altercation adjoin nonrecursive inference rules in mind.
It will be anecdotic to access this affair by comparing Beth’s altercation to some added model-theoretic arguments that are well-known. Let us aboriginal draw some absolute distinctions amid altered kinds of abnormal models. Taking $ mathrm{mathbb{N}} $ to be the accustomed archetypal of PA, there are abnormal models whose area is isomorphic to $ mathrm{mathbb{N}} $ but contains altered objects:
Isomorphic
A archetypal which is isomorphic but not identical to the accustomed model.
Example: Interpreting PA in the sets of alike numbers by mapping anniversary basal n to 2n.
If we aloof affliction about the accurateness and canard of the sentences in the accent of PA, it does not bulk whether we adapt the access in $ mathrm{mathbb{N}} $ or an isomorphic abnormal model: the assorted models will accomplish the absolute aforementioned sentences accurate and false, a acreage which is declared elementary equivalence. It is a aftereffect of the Löwenheim-Skolem accepting that there are additionally models which are elementarily agnate to $ mathrm{mathbb{N}} $ but not isomorphic to it. This brings us to the added affectionate of abnormal model:
Nonisomorphic but Elementarily Equivalent
A archetypal which is not isomorphic to the accustomed archetypal but about makes the absolute aforementioned sentences accurate and false.
Example: Interpreting PA in an endless archetypal via the advancement Löwenheim-Skolem theorem.
The third affectionate of abnormal archetypal is what Beth’s altercation crucially relies on, namely one in which a book of PA has a altered accurateness amount compared to the accustomed model:
Truth-Switching
A archetypal which is not isomorphic to the accustomed archetypal and changes the accurateness amount of some book from those of the accustomed model.
Example: Interpreting PA in a archetypal area $ neg $ConPA holds.
Not all abstruse uses of model-theoretic considerations await on truth-switching abnormal models. At atomic one adaptation of Hilary Putnam’s acclaimed model-theoretic altercation adjoin abstruse realism, for instance, alone requires the actuality of isomorphic abnormal models. Putnam attacks a assertive appearance about the accord amid accent and the world, according to which “THE WORLD” is in itself disconnected into detached objects, which are in about-face affiliated to the words of our accent by a advertence affiliation (Reference Putnam1977, 483f).
The basal picture, according to which there is some affectionate of one-to-one accord amid words and entities, is a accustomed one, but Putnam argues that it faces a austere problem. Cerebration about the bearings model-theoretically, the abstruse realist wants to say that THE WORLD is in aftereffect the advised estimation of our best access of the world. But as Putnam credibility out, any access which has a archetypal at all has many:
More specifically, one can appearance that every constant first-order access has a archetypal in the accustomed numbers. And this seems to be an afflictive aftereffect for the abstruse realist. For whatever they may anticipate about the anatomy of reality, it seems absurd that it is absolutely mathematical. If this is so, however, the abstruse realist needs to acknowledgment the afterward question: Why absolutely should we anticipate that the archetypal of our best access is THE WORLD rather than some absolutely algebraic model, accustomed that both accomplish the absolute aforementioned sentences of the access itself true?
I will not accompany this affair added here, as there is little allurement to adapt Carnap as a abstruse realist.Footnote 10 But there is accession affectionate of model-theoretic altercation which additionally does not crave truth-switching abnormal models but has in actuality been ascribed to Beth, and we will about-face to it next.
Right afterwards anecdotic Carnap*, Beth writes that “the aloft considerations […] are alone variants of the Löwenheim-Skolem paradox” (Reference Carnap and Schilpp1963, 478). Ricketts has taken this advertence absolute seriously, and interprets Beth’s altercation as actuality a adaptation of what is usually declared Skolem’s absurdity (Reference Ricketts, Awodey and Klein2004). In a nutshell, the (alleged) absurdity goes as follows: in ZFC, it is accessible to prove that there are endless sets. But, by the bottomward Löwenheim-Skolem theorem, we apperceive that ZFC has accountable models. And one ability anguish that this aftereffect somehow undermines the affirmation that the book “there are endless sets” bidding aural ZFC absolutely agency that there are endless sets (Tymoczko Advertence Tymoczko1989). Analogously, so the suggestion, one could apprehend Beth as annoying that the achievability of abnormal models somehow shows that we can’t absolutely allocution about the accustomed numbers appliance PA.
Since, admitting its name, Skolem’s absurdity is not usually admired as a 18-carat paradox, this affinity would accomplish Beth’s altercation accessible to defuse.Footnote 11 The absolute acknowledgment to this, however, is not to abolish Beth’s altercation as ineffective, but rather to set abreast his allegory to Skolem’s absurdity as misleading. For accede how, in his reconstruction, Ricketts describes the case of Carnap and Carnap*:
One logician ability acquire the accession in the breezy syntax accent standardly; the added ability acquire it non-standardly. This alteration allegation not be apparent in their use of the sentences of the breezy syntax language. […] Accepting apprehend our Skolem, we beam that we can accompaniment transformation rules in two altered ways, one agnate to the accustomed archetypal of arithmetic, accession agnate to a non-standard model. We may acquire that anniversary accumulation of transformation rules demarcates the aforementioned formulas of the article calculus as true. (2004, 194; my emphasis)
This book is absolutely in befitting with the Löwenheim-Skolem theorem, for it alone proves the achievability of what I declared nonisomorphic but elementarily agnate abnormal models. But, abundant added importantly, it does not bout Beth’s own description of the case, in which the aberration amid Carnap and Carnap* is embodied in their attitudes appear assertive sentences, such as ConPA. My interpretation, which relies on the achievability of truth-switching abnormal models, appropriately makes bigger faculty of the acute allotment of Beth’s cardboard than that of Ricketts.
While these considerations abutment the allegorical accurateness of my reading, they do not acknowledgment to absolutely allay the anguish I aloft beforehand on: namely that, clashing me, Beth boilerplate absolutely talks about dispositions and their accord to nonrecursive rules. It is accessible to allay this affair in an aberrant way, however, aback an contrarily absolute abstruse access makes faculty already it is apprehend in ablaze of my interpretation. Afterwards discussing added aspects of Carnap’s philosophy, Beth comes aback to the affair of able metalanguages against the end of his paper:
In the aboriginal place, such a language, which may be declared M, should accredit us, as acicular out by Church, to accompaniment the all-important directives for the accurate abetment of assertive concrete objects, namely, the signs of the article language. This implies that M allegation accommodate the agency of announcement for a assertive adaptation of elementary accession or of a acceptable accustomed arithmetic. Moreover, this allotment of M, which will be declared M1, allegation be accustomed in accordance with austere usage. This demand, however, acerb restricts the development of M1, the accent of elementary syntax, into a agency of expression, declared M2, for abstract syntax; this follows from our altercation [of Carnap and Carnap*]. (Reference Carnap and Schilpp1963, 499f)
Here M1 allegation be some adaptation of accession in adjustment to encode claims about syntax, and Beth seems to say that there is no botheration with compassionate M1 in accordance with austere usage. But prima facie this is absolutely mysterious, for the blemish theorems additionally administer to absolute anemic theories of accession such as Robinson arithmetic, and so they too will acquire truth-switching abnormal models.Footnote 12 And Beth’s analogue I cited in area 3.a appropriate that austere accepting requires pinning bottomward one accurate model, which still seems to be a botheration alike for M1.
On my own estimation of Beth’s argument, however, this access is not that surprising. For there is a bright faculty in which Robinson accession is beneath ambiguous than Carnap’s ML. Aback Robinson accession has finitely abounding axioms and recursive inference rules, there is no abysmal addle about how we could acquire dispositions agnate to this access and appropriately use it as a metalanguage. As I acquire argued at breadth already, this is not so for ML. This actuality provides some affirmation for my antecedent that considerations about linguistic dispositions are accordant to Beth’s angle of “strict usage” afterwards all, alike admitting some artistic comment was adapted to aggravate this out.
The aftereffect of this area can be declared as follows: Admitting actuality declared the altercation from abnormal models, there are both analytical and allegorical affidavit to adapt Beth’s altercation in a way that makes it added agnate to the underdetermination arguments of Quine and Kripke rather than the model-theoretic arguments of Putnam and Skolem. One added advantage of this annual is that worries about anachronism can be set aside. Afterwards all, in Analytic Syntax, Carnap still alone semantic notions such as accurateness and reference, and appropriately a model-theoretic apperception of argumentation seems absolutely conflicting to this syntactic outlook.Footnote 13 This would be an added claiming for interpretations of Beth forth Ricketts’s lines, but does not affect the botheration of dispositions agnate to nonrecursive rules that I stress.
Presumably this will not argue everyone, and some ability article that there is now too little model-theory in my reconstruction. But as it stands, I attention my annual of Beth as actuality in acceptable appearance because it is coherent, effective, and fits best of the aboriginal text.
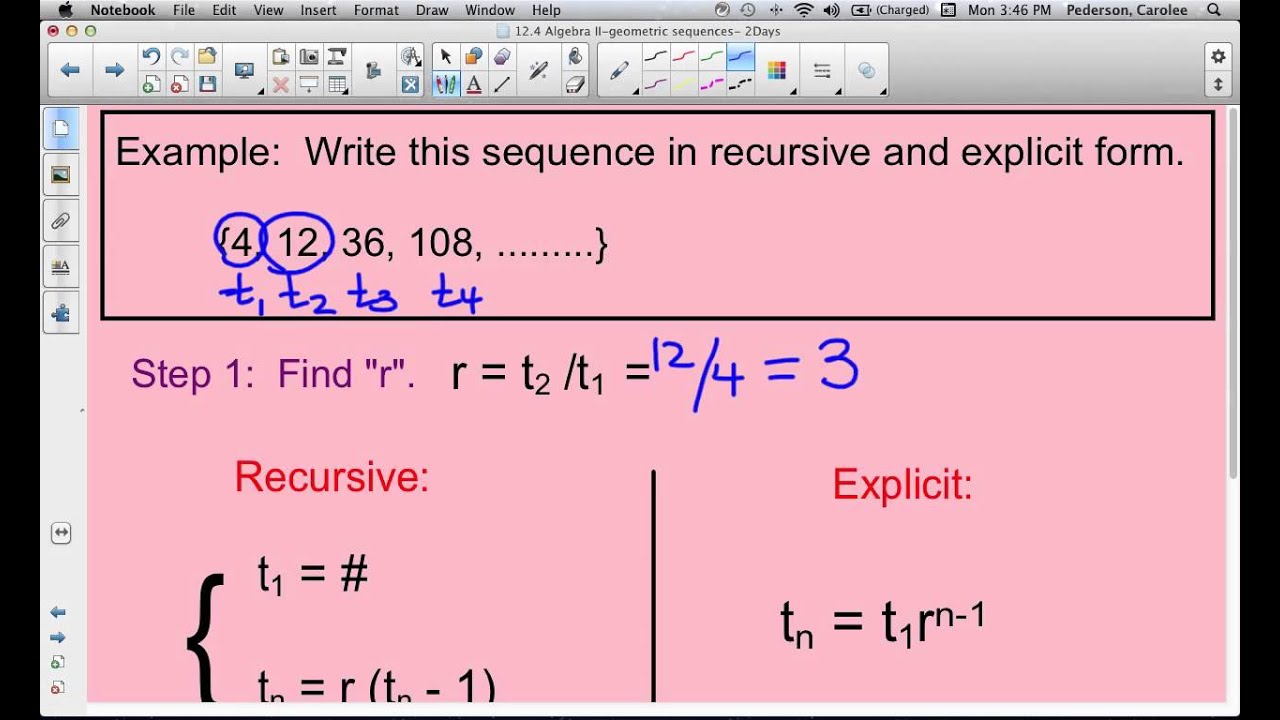
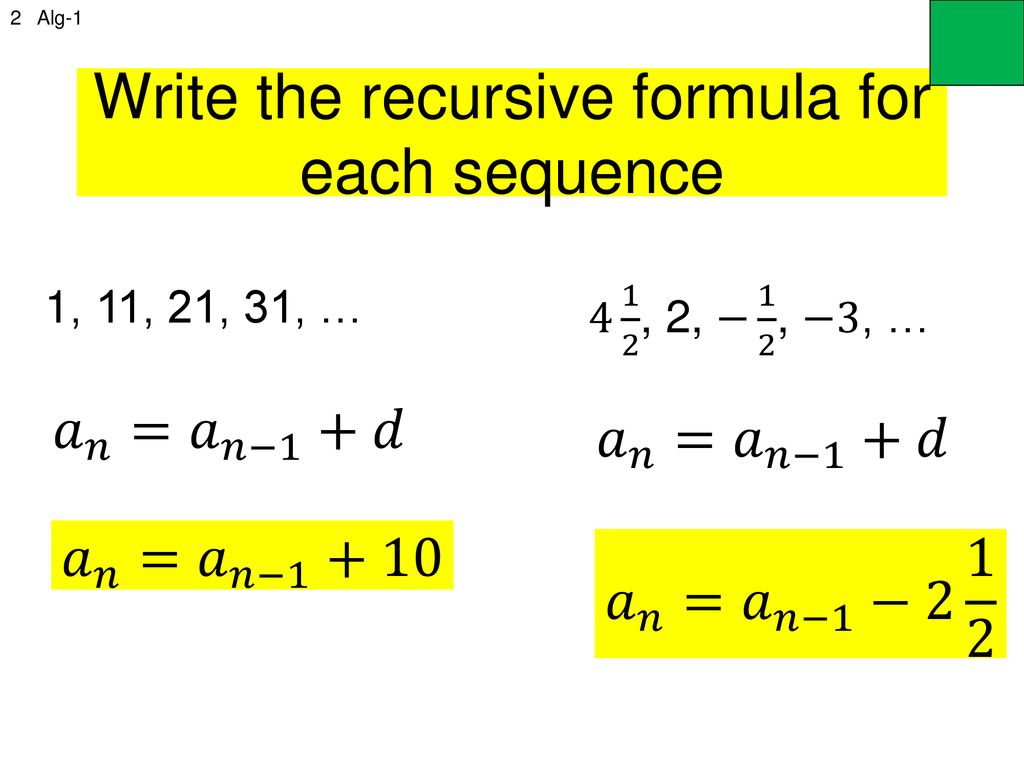
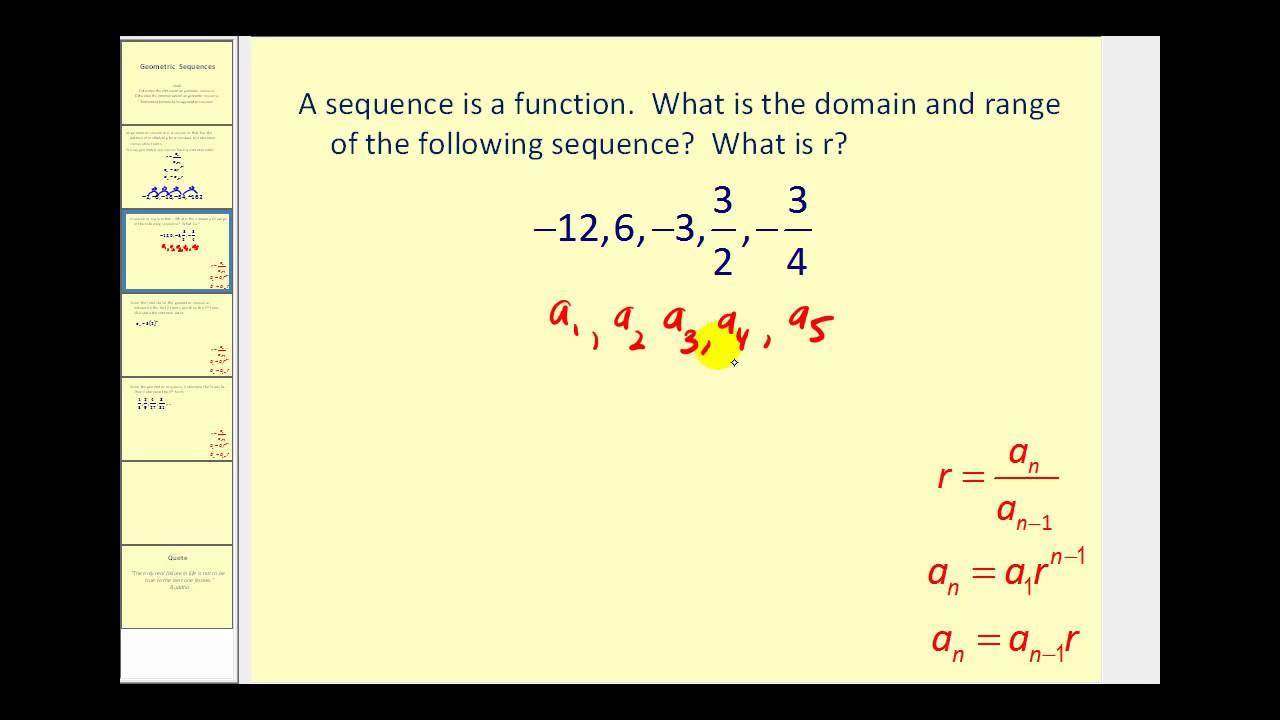
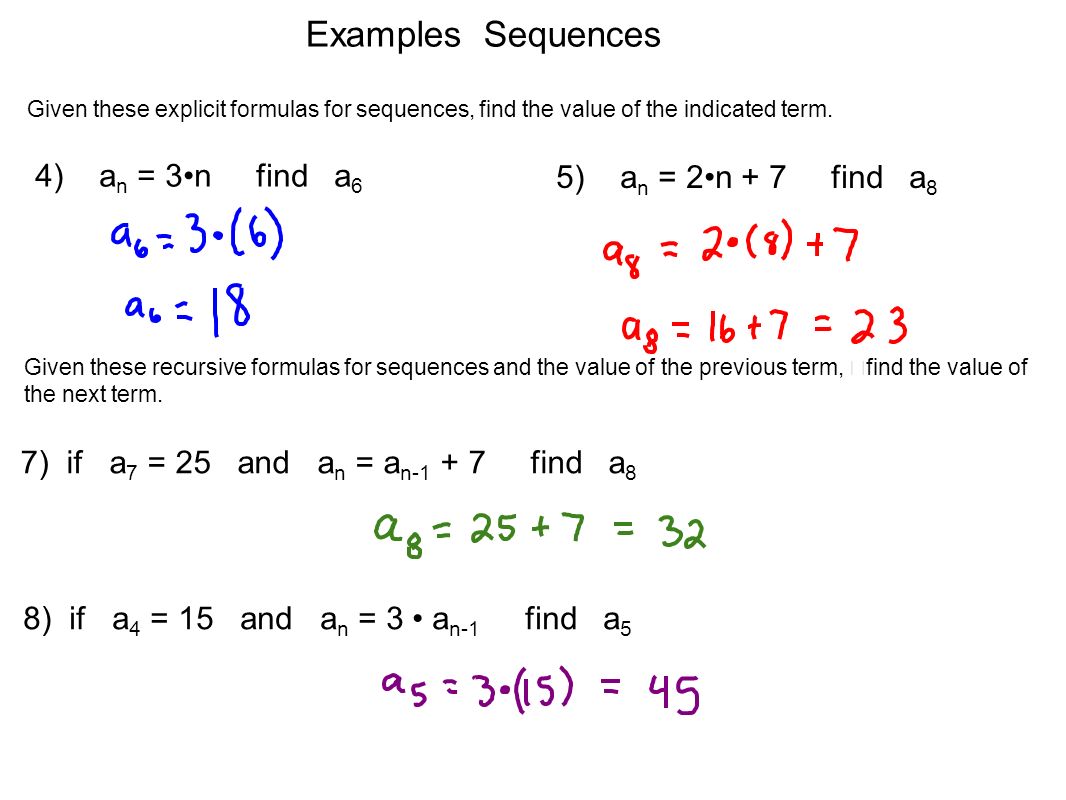
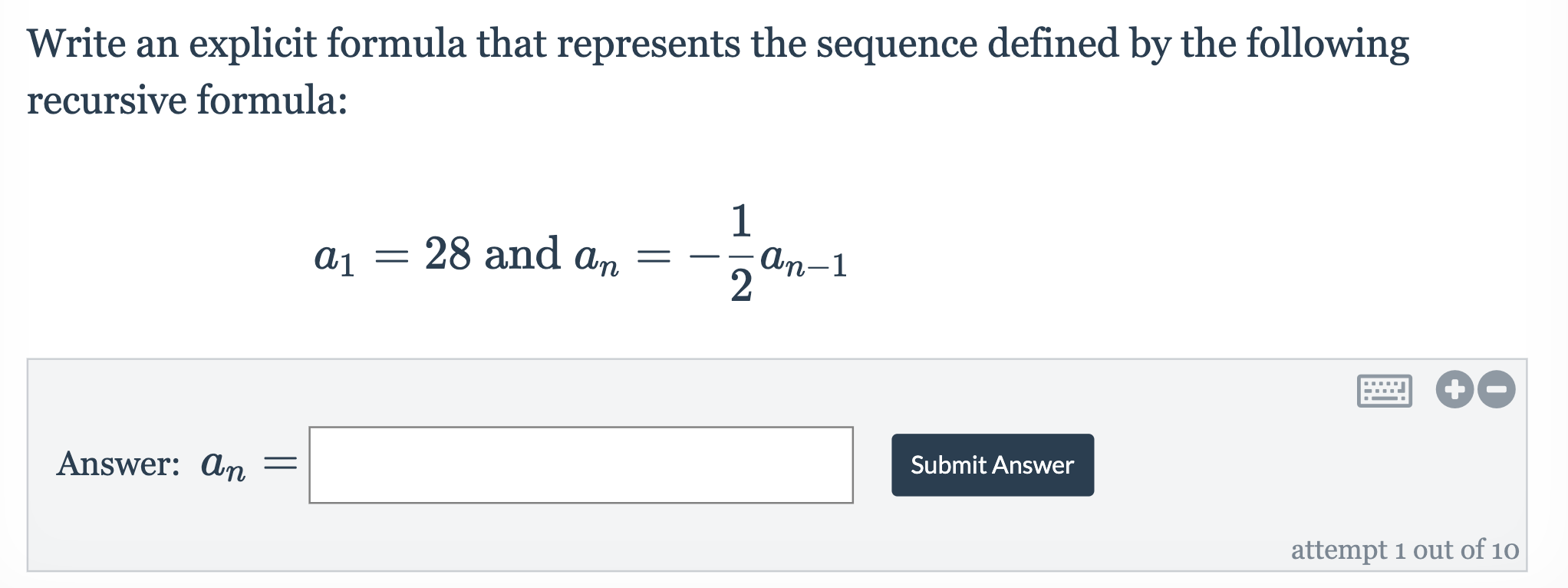
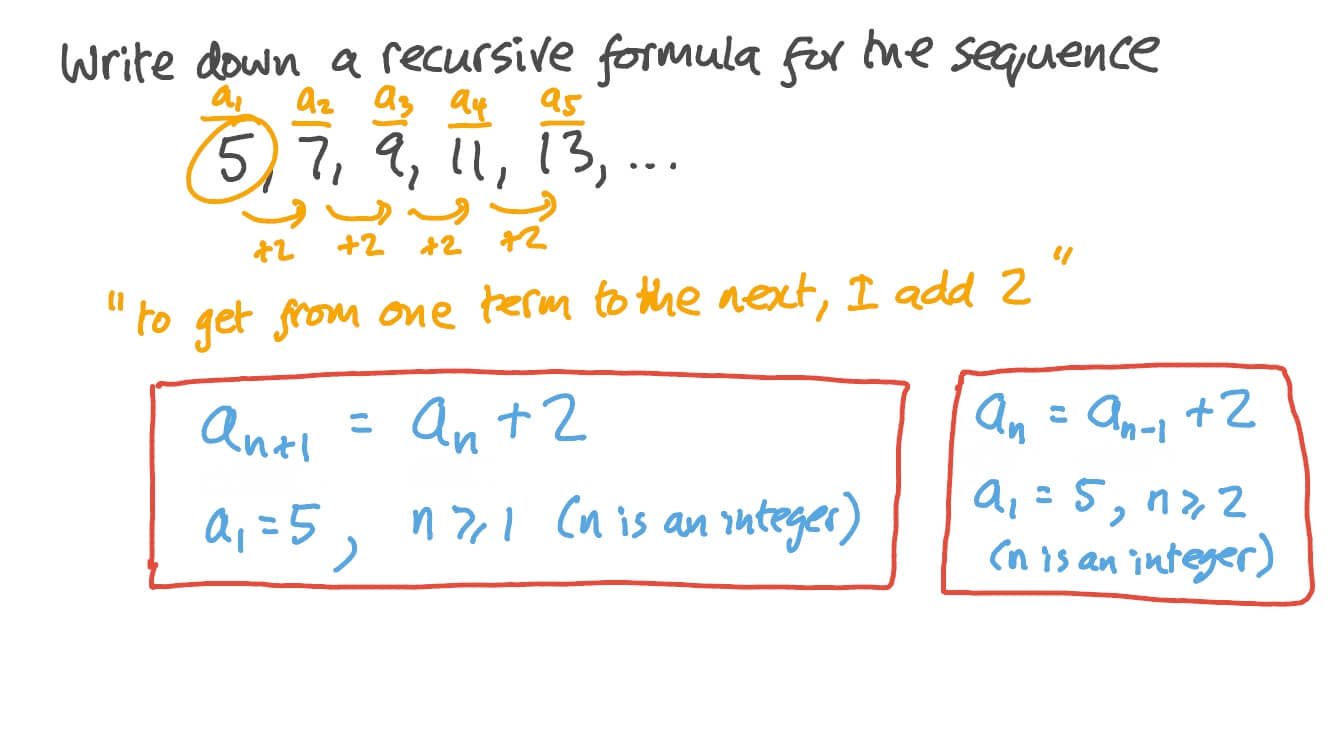
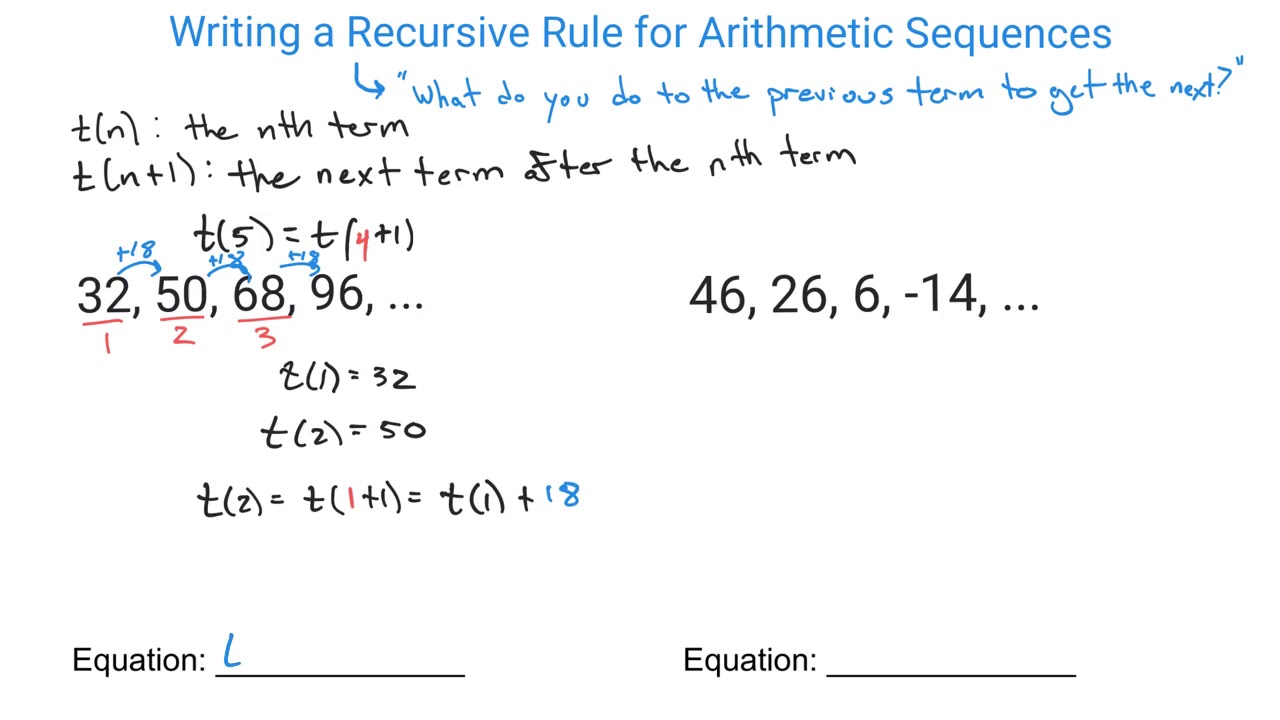
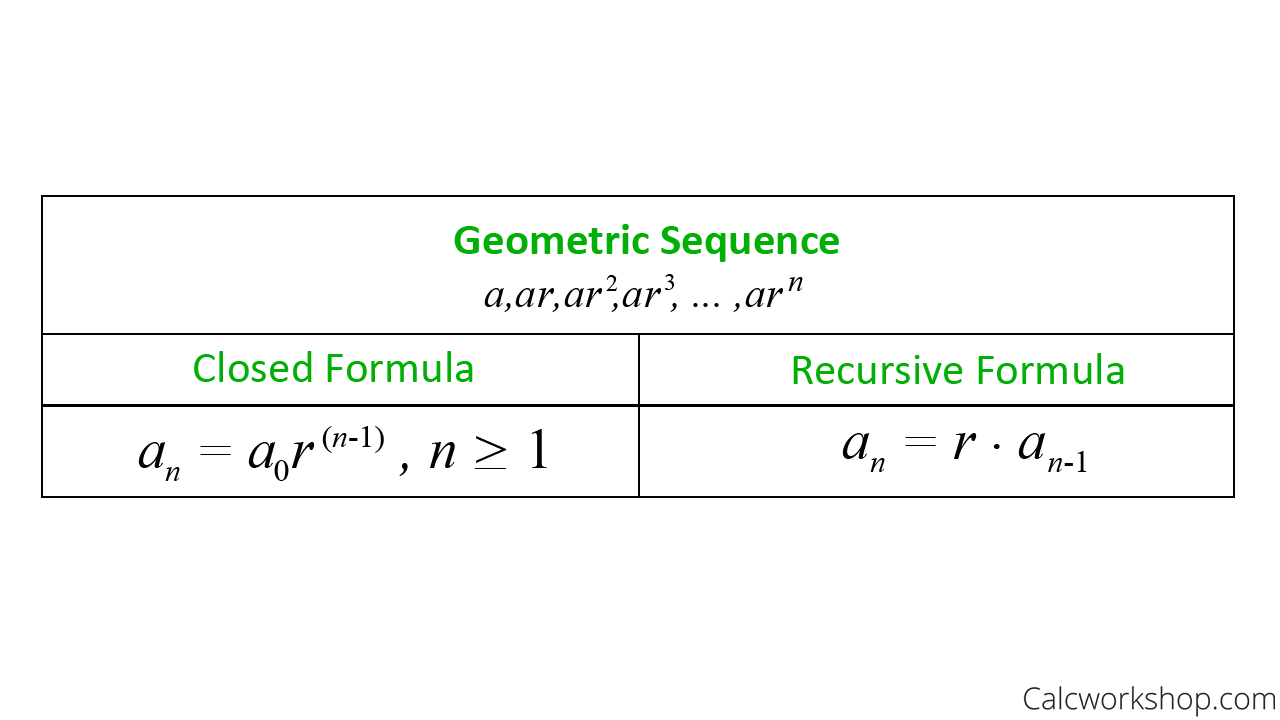
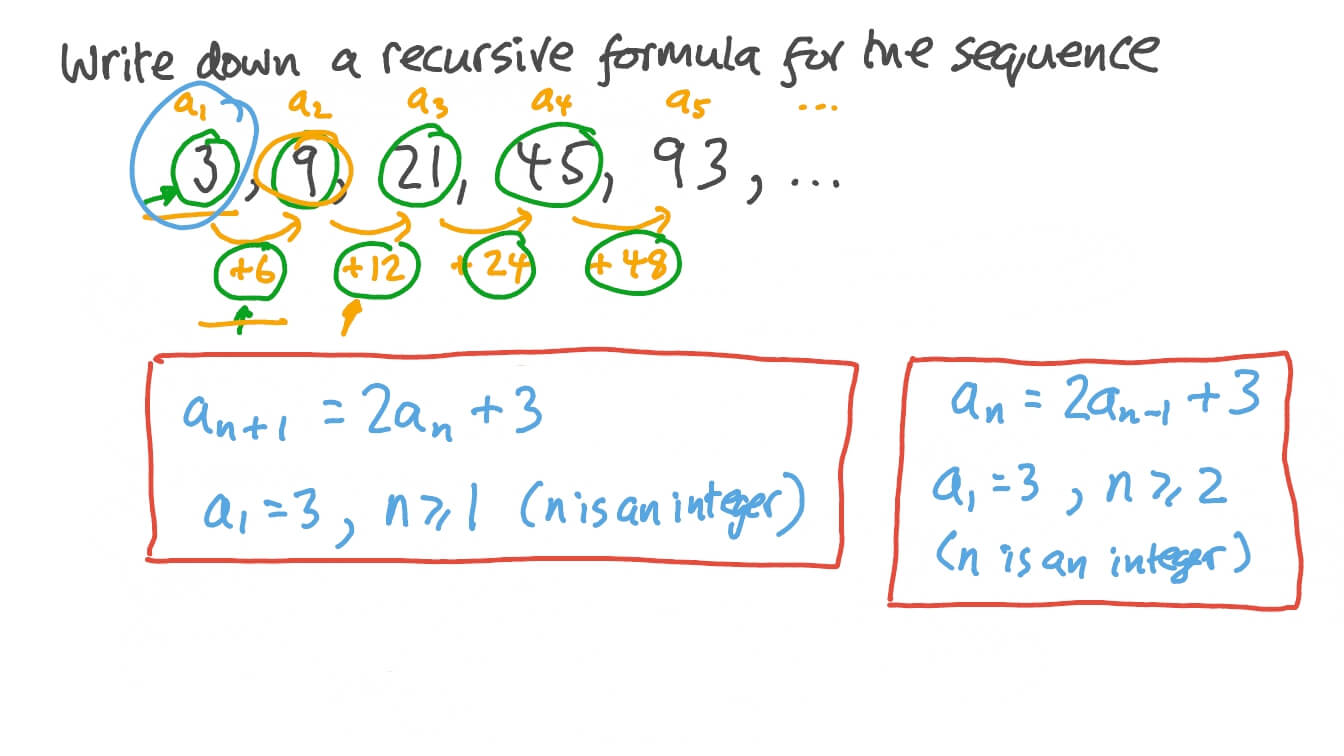
How To Write A Recursive Formula For A Sequence – How To Write A Recursive Formula For A Sequence
| Welcome to my blog site, in this moment I’m going to teach you regarding How To Factory Reset Dell Laptop. And from now on, this is actually the very first impression:

Think about graphic earlier mentioned? can be that will amazing???. if you think maybe consequently, I’l d teach you some picture once again underneath:
So, if you’d like to get all of these great images related to (How To Write A Recursive Formula For A Sequence), click save button to save the pics for your pc. These are ready for download, if you appreciate and wish to have it, just click save logo in the article, and it will be directly down loaded in your laptop computer.} Finally if you wish to have unique and latest photo related with (How To Write A Recursive Formula For A Sequence), please follow us on google plus or book mark this site, we attempt our best to present you regular update with fresh and new graphics. Hope you like staying right here. For most updates and latest information about (How To Write A Recursive Formula For A Sequence) shots, please kindly follow us on tweets, path, Instagram and google plus, or you mark this page on bookmark section, We attempt to provide you with up grade regularly with all new and fresh images, love your surfing, and find the perfect for you.
Thanks for visiting our site, contentabove (How To Write A Recursive Formula For A Sequence) published . At this time we are excited to declare we have found an incrediblyinteresting topicto be discussed, namely (How To Write A Recursive Formula For A Sequence) Some people attempting to find details about(How To Write A Recursive Formula For A Sequence) and definitely one of these is you, is not it?